Question
A cylinder and a cone have congruent bases and heights. _____________ will be the relationship of the volumes of the two figures.
- The volume of the cylinder will be twice the volume of the cone.
- The volume of the cone will be one-fourth the volume of the cylinder.
- The volume of the cylinder will be 4/3 the volume of the cone.
- The volume of the cylinder will be three times the volume of the cone.

When it comes to Mensuration one can find an expression for an volume of a structure and find the required attributes of a structure from the given data using substitution method. One can find Volume expression using general volume calculation using area and height or through integrating a small unit to form a cone.
Calculate volume of cylinder and volume of cone then, relate between them.
The correct answer is: The volume of the cylinder will be three times the volume of the cone.
Assumption:
Let, radius and height of the cylinder and cone are equal.
Since, we have derived volume of cylinder as  and volume of cone
and volume of cone  .
.
Hence,  =
= 
 = 3;
= 3;
***Volume of the cylinder = 3 Volume of the cone
Volume of the cone
Volume of the cylinder is three times the volume of the cone.
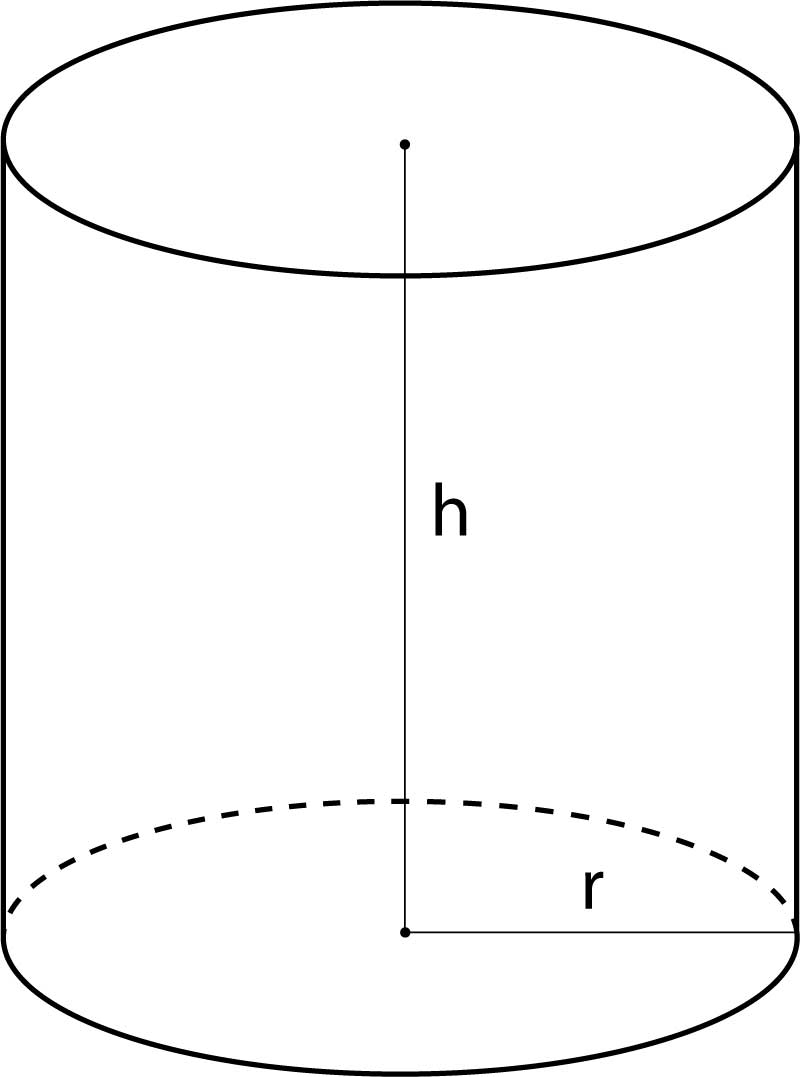

Cylinder and cone are the 3-Dimensional figures where cylinder resides between two circle and cone resides on one circle. Let us assume height of a cylinder and cone is h and radius of a base of cylinder and cone is r. Then,
Volume of any Structure = Base Area Height
Hence, Volume of the Cylinder = .
And, we know that Volume of the Cone is 1/3 of the Volume of the cylinder
Hence, Volume of the Cone = .
Related Questions to study
A cone has the radius of 2 inches and the height of 4 inches. Find the volume of the cone. Round your answer to the nearest tenth.
Since, we have derived an expression for volume of a cone as . Substitute the given attributes to find the volume of a cone.
Given that:
Radius of base of a cone = 2 inches
Height of a cone = 4 inches
Hence, Volume of a cone =
=
=16.7467 inches3.
*** Volume of a cone is 16.7567 ~ 16.76 cubic inches.
A cone has the radius of 2 inches and the height of 4 inches. Find the volume of the cone. Round your answer to the nearest tenth.
Since, we have derived an expression for volume of a cone as . Substitute the given attributes to find the volume of a cone.
Given that:
Radius of base of a cone = 2 inches
Height of a cone = 4 inches
Hence, Volume of a cone =
=
=16.7467 inches3.
*** Volume of a cone is 16.7567 ~ 16.76 cubic inches.
A cone has the radius of 10 inches and the height of 9 inches. Find the volume of the cone. Round your answer to the nearest tenth.
Since, we have derived an expression for volume of a cone as . Hence, calculate any attribute of a structure using the given data.
Given that:
Radius of a cone : 10 inches
Height of a cone : 9 inches
Hence, the volume of a cone =
=
=942.4876 inches3.
Hence, volume of a cone is 942.4876 ~ 942.49 cubic inches.
A cone has the radius of 10 inches and the height of 9 inches. Find the volume of the cone. Round your answer to the nearest tenth.
Since, we have derived an expression for volume of a cone as . Hence, calculate any attribute of a structure using the given data.
Given that:
Radius of a cone : 10 inches
Height of a cone : 9 inches
Hence, the volume of a cone =
=
=942.4876 inches3.
Hence, volume of a cone is 942.4876 ~ 942.49 cubic inches.
A cone has the radius of 6 inches and the height of 5 inches. Find the volume of the cone. Round your answer to the nearest tenth.
Since, we have derived an expression for volume of a cone as r2h. Hence, just substitute values of a given attributes to find the volume of a cone.
Given that:
Radius of base of a cone = 6 inches
Height of base of a cone = 5 inches
Hence, Volume of a cone = r2h
=
=188.49 inches3.
***Volume of a cone is 188.49 cubic inches.
A cone has the radius of 6 inches and the height of 5 inches. Find the volume of the cone. Round your answer to the nearest tenth.
Since, we have derived an expression for volume of a cone as r2h. Hence, just substitute values of a given attributes to find the volume of a cone.
Given that:
Radius of base of a cone = 6 inches
Height of base of a cone = 5 inches
Hence, Volume of a cone = r2h
=
=188.49 inches3.
***Volume of a cone is 188.49 cubic inches.
Find the volume of corn held in this cone-shaped grain silo. Use 3.14 for π and round to the nearest cubic foot.

Since, we have derived an expression for volume of a cone as . hence, substituting the values of radius and slant height values we can obtain volume of a cone easily.
Given that:
Slant height of a cone (l) = 10 feet
Radius of a cone (r) = 5 feet
Hence, volume of a cone is =
=
=
= 301.44 feet3.
Hence, Volume of a cone is 301.44 ~ 301 cubic feet.
Find the volume of corn held in this cone-shaped grain silo. Use 3.14 for π and round to the nearest cubic foot.

Since, we have derived an expression for volume of a cone as . hence, substituting the values of radius and slant height values we can obtain volume of a cone easily.
Given that:
Slant height of a cone (l) = 10 feet
Radius of a cone (r) = 5 feet
Hence, volume of a cone is =
=
=
= 301.44 feet3.
Hence, Volume of a cone is 301.44 ~ 301 cubic feet.
A cone has the radius of 5.5 inches and the height of 12 inches. Find the volume of the cone. Round your answer to the nearest tenth.
Since, we have derived the volume of the cone as r2h. Then, just substitute the given values and find the volume of the cone.
Given Data:
Radius of the cone = 5.5 inches
Height of the cone = 12 inches
Hence, Volume of the Cone = r2h
=
=379.64
***Volume of the cone is 379.64.. ~ 380.13 inches3.
A cone has the radius of 5.5 inches and the height of 12 inches. Find the volume of the cone. Round your answer to the nearest tenth.
Since, we have derived the volume of the cone as r2h. Then, just substitute the given values and find the volume of the cone.
Given Data:
Radius of the cone = 5.5 inches
Height of the cone = 12 inches
Hence, Volume of the Cone = r2h
=
=379.64
***Volume of the cone is 379.64.. ~ 380.13 inches3.
Calculate the volume of a cone with a diameter of 30 feet and a height of 60 feet. Use 3.14 for π.
Since, we have derived the volume of the cone it is easy to find the volume from the given data.
Given That:
Diameter of a base of a cone = 30 feet
we know that 2Radius=Diameter then,
Radius of a base of a cone =15 feet
Height of a cone = 60 feet
From the volume of the cone = r2h
=
=14130 feet3
***Hence, the volume of a cone is 14130 cubic feet.
Calculate the volume of a cone with a diameter of 30 feet and a height of 60 feet. Use 3.14 for π.
Since, we have derived the volume of the cone it is easy to find the volume from the given data.
Given That:
Diameter of a base of a cone = 30 feet
we know that 2Radius=Diameter then,
Radius of a base of a cone =15 feet
Height of a cone = 60 feet
From the volume of the cone = r2h
=
=14130 feet3
***Hence, the volume of a cone is 14130 cubic feet.
A cone has the radius of 2 cm and the height of 3 cm. Calculate the volume of the cone. Round your answer to the nearest tenth.
Since, we have derived the volume of a cone as r2h.
Given that:
Radius of a base of a cone : 2 cm
Height of a cone : 3 cm
Hence, Volume of a cone = r2h
=
=12.571428 cubic cm.
* it may vary by taking different values(3.14 for now).
**** Hence, the volume of a cone is 12.57(rounding off to it's nearest tenth).
A cone has the radius of 2 cm and the height of 3 cm. Calculate the volume of the cone. Round your answer to the nearest tenth.
Since, we have derived the volume of a cone as r2h.
Given that:
Radius of a base of a cone : 2 cm
Height of a cone : 3 cm
Hence, Volume of a cone = r2h
=
=12.571428 cubic cm.
* it may vary by taking different values(3.14 for now).
**** Hence, the volume of a cone is 12.57(rounding off to it's nearest tenth).
A cone has the radius of 10 cm and the height of 8 cm. Find the volume of the cone. Round your answer to the nearest tenth.
Hence, We have derived an expression for an volume of the cone as r2h.
Given that:
radius of the base of a cone: 10 cm
height of a cone : 8 cm
Hence, Volume of a cone= r2h.
=
= 837.334 cubic cm
*** Volume of the con is 837.334 cubic cm.
* It may vary based on the value of taken.(In general
=3.14)
A cone has the radius of 10 cm and the height of 8 cm. Find the volume of the cone. Round your answer to the nearest tenth.
Hence, We have derived an expression for an volume of the cone as r2h.
Given that:
radius of the base of a cone: 10 cm
height of a cone : 8 cm
Hence, Volume of a cone= r2h.
=
= 837.334 cubic cm
*** Volume of the con is 837.334 cubic cm.
* It may vary based on the value of taken.(In general
=3.14)
A cone with a radius of 5 inches has a volume of approximately 314 cubic inches. Which of the following best represents the height of the cone?
Since, We have derived volume of a cone as r2h.
Given that:
Radius of base = 5 inches
Volume of a cone = 314 cubic inches
*** From the formula of volume of a cone that we derived, height of a cone h can be retrieved as
h=
Height =
= 12 inches
*** Height of a cone is 12 inches.
A cone with a radius of 5 inches has a volume of approximately 314 cubic inches. Which of the following best represents the height of the cone?
Since, We have derived volume of a cone as r2h.
Given that:
Radius of base = 5 inches
Volume of a cone = 314 cubic inches
*** From the formula of volume of a cone that we derived, height of a cone h can be retrieved as
h=
Height =
= 12 inches
*** Height of a cone is 12 inches.
An ice cream cone that is 4 inches tall has an opening that is 8 inches across. Find the volume of the cone.
Hence, We have derived the volume of a cone that is r2h.
Given That:
Height of the Cone (h) = 4 inches
Diameter of the Base = 8 inches
We know in any Circle:
2Radius=Diameter
Then, Radius=4 inches.
Hence, Base area = r2
=3.14
16
= 16.7467
Hence, The volume of a cone = Base Area Height
=16.74674
=66.9868 ~67.02
Volume of Cone is 67.02 cubic inches.
An ice cream cone that is 4 inches tall has an opening that is 8 inches across. Find the volume of the cone.
Hence, We have derived the volume of a cone that is r2h.
Given That:
Height of the Cone (h) = 4 inches
Diameter of the Base = 8 inches
We know in any Circle:
2Radius=Diameter
Then, Radius=4 inches.
Hence, Base area = r2
=3.14
16
= 16.7467
Hence, The volume of a cone = Base Area Height
=16.74674
=66.9868 ~67.02
Volume of Cone is 67.02 cubic inches.
A cone and a cylinder have the same diameter and height. How many times greater is the cylinder's volume than the cone's?

Since, We know the volume of a cylinder and volume of a cone, we can easily relate between them.
As, we seen before Volume of a Cylinder is given by : r2h
Similarly, Volume of the Cone is: r2h.
***Volume of a Cone = Volume of a Cylinder.
***Volume of a Cylinder = 3 Volume of Cone.
Hence, We can say that Volume of the Cylinder is Three times the volume of the Cone.
A cone and a cylinder have the same diameter and height. How many times greater is the cylinder's volume than the cone's?

Since, We know the volume of a cylinder and volume of a cone, we can easily relate between them.
As, we seen before Volume of a Cylinder is given by : r2h
Similarly, Volume of the Cone is: r2h.
***Volume of a Cone = Volume of a Cylinder.
***Volume of a Cylinder = 3 Volume of Cone.
Hence, We can say that Volume of the Cylinder is Three times the volume of the Cone.
Mike created a cone that has a diameter of 8 feet and a height of 3 feet. Find the volume of this cone.
The Given Data:
Diameter of a circular base: 8 feet
We know 2radius=diameter. Then. radius=4 feet.
height of a cone = 3 feet.
Hence, Volume of a cone = Area of a circular base
Height
Area of a circular base: r2 = 3.14
42 = 50.24 ft2 .
Volume of Cone = 50.24
3 = 50.24 ft3.
Hence, The volume of a cone is equal to the 50.24 cubic feet.
Mike created a cone that has a diameter of 8 feet and a height of 3 feet. Find the volume of this cone.
The Given Data:
Diameter of a circular base: 8 feet
We know 2radius=diameter. Then. radius=4 feet.
height of a cone = 3 feet.
Hence, Volume of a cone = Area of a circular base
Height
Area of a circular base: r2 = 3.14
42 = 50.24 ft2 .
Volume of Cone = 50.24
3 = 50.24 ft3.
Hence, The volume of a cone is equal to the 50.24 cubic feet.



