Maths-
General
Easy
Question
Domain of definition of the function
, is
Hint:
Wavy curve method
The wavy curve method (also called the method of intervals) is a strategy used to solve inequalities of the form 
.
The method uses the fact that 
,can only change sign at its zeroes and vertical asymptotes, so we can use the roots of 
and to sketch a graph of the function over different intervals.
The correct answer is:
Given, ,
....... (1)
x(x-1)(x+1) > 0
By wavy curve method
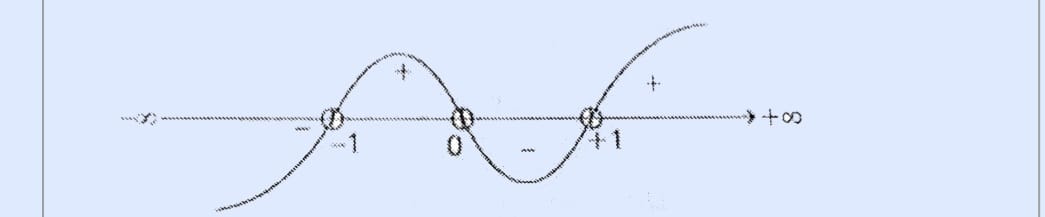
When x> 1, function is positive
When x lies between 0 and 1, function is negative
When x lies between -1 and 0, function is positive
When x lies between < -1 , function is negative
Related Questions to study
physics-
If 
represent the work done in moving a particle from A to B along three different paths 1, 2 and 3 respectively(as shown)in the gravitational field of a point mass m. Find the correct relation between 
If represent the work done in moving a particle from A to B along three different paths 1, 2 and 3 respectively(as shown)in the gravitational field of a point mass m. Find the correct relation between
physics-General
physics-
Given below is a graph between a variable force 
(along 
-axis) and the displacement 
(along 
-axis) of a particle in one dimension. The work done by the force in the displacement interval between 
and 
is
Given below is a graph between a variable force (along -axis) and the displacement (along -axis) of a particle in one dimension. The work done by the force in the displacement interval between and is
physics-General
physics-
A particle of mass 
moving with a velocity 
makes an elastic one dimensional collision with a stationary particle of mass establishing a contact with it for extremely small time 
. Their force of contact increases from zero to 
linearly in time 
, remains constant for a further time 
and decreases linearly from to zero in further time as shown. The magnitude possessed by is
A particle of mass moving with a velocity makes an elastic one dimensional collision with a stationary particle of mass establishing a contact with it for extremely small time . Their force of contact increases from zero to linearly in time , remains constant for a further time and decreases linearly from to zero in further time as shown. The magnitude possessed by is
physics-General
Maths-
Let 
be a set containing 10 distinct elements, then the total number of distinct functions from to is-
The domain is defined as the set which is to input in a function. We say that input values satisfy a function. The range is defined as the actual output supposed to be obtained by entering the domain of the function.
Co-domain is defined as the values that are present in the right set that is set Y, possible values expected to come out after entering domain values.
Let be a set containing 10 distinct elements, then the total number of distinct functions from to is-
Maths-General
The domain is defined as the set which is to input in a function. We say that input values satisfy a function. The range is defined as the actual output supposed to be obtained by entering the domain of the function.
Co-domain is defined as the values that are present in the right set that is set Y, possible values expected to come out after entering domain values.
maths-
The number of bijective functions from set A to itself when a contains 106 elements-
The number of bijective functions from set A to itself when a contains 106 elements-
maths-General
physics-
The work done by a force acting on a body is as shown in the graph. The total work done in covering an initial distance of 
is
The work done by a force acting on a body is as shown in the graph. The total work done in covering an initial distance of is
physics-General
physics-
A particle is acted upon by a force 
which varies with position as shown in figure. If the particle at 
has kinetic energy of 25 J, then the kinetic energy of the particle at 
is
A particle is acted upon by a force which varies with position as shown in figure. If the particle at has kinetic energy of 25 J, then the kinetic energy of the particle at is
physics-General
physics-
A vertical spring with force constant 
is fixed on a table. A ball of mass at a height 
above the free upper end of the spring falls vertically on the spring so that the spring is compressed by a distance 
. The net work done in the process is
A vertical spring with force constant is fixed on a table. A ball of mass at a height above the free upper end of the spring falls vertically on the spring so that the spring is compressed by a distance . The net work done in the process is
physics-General
physics-
Figure shows the -graph. Where is the force applied and is the distance covered
By the body along a straight line path. Given that is in 
and 
in 
, what is the work done?
Figure shows the -graph. Where is the force applied and is the distance covered
By the body along a straight line path. Given that is in and in , what is the work done?
physics-General
maths-
Range of function f(x) = 
, 
is given by -
Range of function f(x) = , is given by -
maths-General
physics-
A light inextensible string that goes over a smooth fixed pulley as shown in the figure connects two blocks of masses 
and 
. Taking 
, find the work done (in joules) by the string on the block of mass during the first second after the system is released from rest
A light inextensible string that goes over a smooth fixed pulley as shown in the figure connects two blocks of masses and . Taking , find the work done (in joules) by the string on the block of mass during the first second after the system is released from rest
physics-General
physics-
An object of mass is tied to a string of length 
and a variable horizontal force is applied on it which starts at zero and gradually increases until the string makes an angel 
with the vertical. Work done by the force is
An object of mass is tied to a string of length and a variable horizontal force is applied on it which starts at zero and gradually increases until the string makes an angel with the vertical. Work done by the force is
physics-General
physics-
A block of mass 
kg sliding on a smooth horizontal surface with a velocity 
meets the spring of spring constant 
fixed at one end as shown in figure. The maximum compression of the spring and velocity of block as is returns to the original position respectively are
A block of mass kg sliding on a smooth horizontal surface with a velocity meets the spring of spring constant fixed at one end as shown in figure. The maximum compression of the spring and velocity of block as is returns to the original position respectively are
physics-General
physics-
The relationship between the force F and position of a body is as shown in figure. The work done in displacing the body from 
to 
m will be
The relationship between the force F and position of a body is as shown in figure. The work done in displacing the body from to m will be
physics-General
physics-
Three objects 
and 
are kept in a straight line on a frictionless horizontal surface. These have masses 
and respectively. The object moves towards 
with a speed 
and makes an elastic collision with it. Thereafter, makes completely inelastic collision with . All motions occur on the same straight line. Find the final speed (in 
) of the object
Three objects and are kept in a straight line on a frictionless horizontal surface. These have masses and respectively. The object moves towards with a speed and makes an elastic collision with it. Thereafter, makes completely inelastic collision with . All motions occur on the same straight line. Find the final speed (in ) of the object
physics-General
