Maths-
General
Easy
Question
- 1
- 0
- a
none of these
none of these
Hint:
We can apply L'Hopital's rule, also commonly spelled L'Hospital's rule, whenever direct substitution of a limit yields an indeterminate form. This means that the limit of a quotient of functions (i.e., an algebraic fraction) is equal to the limit of their derivatives.
In this question, we have to find value of 
.
The correct answer is: a
We first try substitution:
= 
= 
Since the limit is in the form 0 over 0. It is indeterminate—we don’t yet know what is it. We need to do some work to put it in a form where we can determine the limit.
( L'Hopital's Rule for zero over zero, 
= 
)
(d(sinax)/dx = a cos(ax) , -sin x)
On substituting, We get
= a
We can only apply the L’Hospital’s rule if the direct substitution returns an indeterminate form, that means 
Related Questions to study
maths-
maths-General
Maths-
Maths-General
maths-
maths-General
maths-
If 
then xy+1=
If then xy+1=
maths-General
physics-
A wire of length 2 m is made from 10 cm3 of copper. A force F is applied so that its length increases by 2 mm. Another wire of length 8 m is made from the same volume of copper. If the force F is applied to it, its length will increase by….
A wire of length 2 m is made from 10 cm3 of copper. A force F is applied so that its length increases by 2 mm. Another wire of length 8 m is made from the same volume of copper. If the force F is applied to it, its length will increase by….
physics-General
Maths-
The absolute value (or modulus) | x | of a real number x is the non-negative value of x without regard to its sign.
Maths-General
The absolute value (or modulus) | x | of a real number x is the non-negative value of x without regard to its sign.
maths-
The velocity of a train which starts from rest is given by
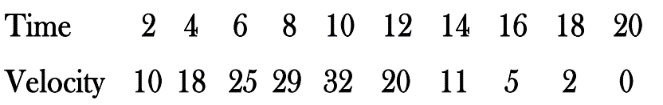
The time being recorded in minutes from the start and the speed in kmph. Find approximately the total distance run in 20 minutes by simpson’s rule is
The velocity of a train which starts from rest is given by
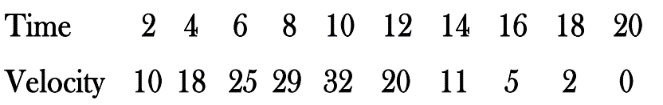
The time being recorded in minutes from the start and the speed in kmph. Find approximately the total distance run in 20 minutes by simpson’s rule is
maths-General
maths-
A river is 40 metre wide. The depth d in metres at a distance x metres from a bank is given in the following table. Using simpson's rule, estimate the area of cross section of the river in square metres is
A river is 40 metre wide. The depth d in metres at a distance x metres from a bank is given in the following table. Using simpson's rule, estimate the area of cross section of the river in square metres is
maths-General
physics-
The refractive index of the material of the prism and liquid are 1.56 and 1.32 respectively. What will be the value of 
for the following refraction
The refractive index of the material of the prism and liquid are 1.56 and 1.32 respectively. What will be the value of for the following refraction
physics-General
physics-
An isosceles prism of angle 120° has a refractive index of 1.44. Two parallel monochromatic rays enter the prism parallel to each other in air as shown. The rays emerging from the opposite faces
An isosceles prism of angle 120° has a refractive index of 1.44. Two parallel monochromatic rays enter the prism parallel to each other in air as shown. The rays emerging from the opposite faces
physics-General
physics-
If the interatomic spacing in a steel wire is 
the force constant =…..
If the interatomic spacing in a steel wire is the force constant =…..
physics-General
maths-
maths-General
maths-
In the figure rectangle ABCD, what is the probability that a randomly chosen point that in ABCD lies inside the triangle ABE is 
In the figure rectangle ABCD, what is the probability that a randomly chosen point that in ABCD lies inside the triangle ABE is
maths-General
physics-
Which one of the following spherical lenses does not exhibit dispersion? The radii of curvature of the surfaces of the lenses are as given in the diagrams
Which one of the following spherical lenses does not exhibit dispersion? The radii of curvature of the surfaces of the lenses are as given in the diagrams
physics-General
physics-
Shown in the figure here is a convergent lens placed inside a cell filled with a liquid. The lens has focal length + 20 cm when in air and its material has refractive index 1.50. If the liquid has refractive index 1.60, the focal length of the system is
Shown in the figure here is a convergent lens placed inside a cell filled with a liquid. The lens has focal length + 20 cm when in air and its material has refractive index 1.50. If the liquid has refractive index 1.60, the focal length of the system is
physics-General
