Have a query? Ask a Tutor!!

Access to best educators and exclusive paper discussions

Offsite Campus Turito Championship

Can’t find what you’re looking for?

Our tutors are from top schools around the world, and they are waiting for you 24/7, anytime, anywhere.

Homework- One to One Solutions
Understand concepts to solve better
Get your doubts solved instantly

Maths-

General

Easy

Question

The derivative of even function is

Even function
Odd function
Cannot be definitely known
Even function or odd function

hint Hint:

We are asked the derivative of an even function. We will see the definition of even function first. Then we will find it's derivative.

The correct answer is: Odd function

Let f(x) be any function.
f(x) is said to be an even function if it statisfy the given condition.
f(x) = f(-x) ...(1)
It means if the input is negative or positive we will get the same output.
f(x) is said to be an odd function if it satisfy the given condition.
f(-x) = -f(x) ...(2)
Now, we will take the derivative of equation (1)
f'(x) = -f'(-x)
We can see that the derivative of even function satisfy the condition of an odd function.
So, the derivative of an even function is an odd function.

For such questions, we should know the definition of even and odd function.

Related Questions to study

If $R rightwards arrow R$ $d i f i n e d blank b y blank f open parentheses x close parentheses equals x minus open square brackets x close square brackets left parenthesis w h e r e$ [x] is the greatest integer not exceeding (x) then the set of discontinuity of f is...

If $R rightwards arrow R$ $d i f i n e d blank b y blank f open parentheses x close parentheses equals x minus open square brackets x close square brackets left parenthesis w h e r e$ [x] is the greatest integer not exceeding (x) then the set of discontinuity of f is...

$f open parentheses x close parentheses equals open curly brackets table row cell open square brackets x close square brackets blank i f minus 3 less than x less or equal than negative 1 end cell row cell open vertical bar x close vertical bar blank i f minus 1 less than x less than 1 end cell row cell open vertical bar left square bracket negative x right square bracket close vertical bar blank i f blank 1 less or equal than x less than 3 end cell end table close$ then {x/f(x) $greater or equal than 0$ }=

$f open parentheses x close parentheses equals open curly brackets table row cell open square brackets x close square brackets blank i f minus 3 less than x less or equal than negative 1 end cell row cell open vertical bar x close vertical bar blank i f minus 1 less than x less than 1 end cell row cell open vertical bar left square bracket negative x right square bracket close vertical bar blank i f blank 1 less or equal than x less than 3 end cell end table close$ then {x/f(x) $greater or equal than 0$ }=

The order of reactivity of following alcohols, towards conc. HCl is:
i)
ii)
iii)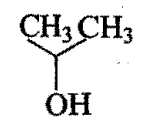
iv)

The order of reactivity of following alcohols, towards conc. HCl is:
i)
ii)
iii)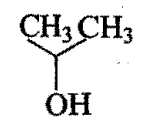
iv)

Chemistry-General

parallel

In the following compounds piperidine
I) pyridine
II) morpho line
III) and pyrrole
IV) the order of basicity is:

In the following compounds piperidine
I) pyridine
II) morpho line
III) and pyrrole
IV) the order of basicity is:

Chemistry-General

If $blank f blank open square brackets x close square brackets$ denotes the greatestinterger not exceeding x and if the function f defined by $blank f open parentheses x close parentheses equals open curly brackets table row cell fraction numerator a plus 2 blank c o s blank x over denominator x to the power of 2 end exponent end fraction blank left parenthesis x less than 0 right parenthesis end cell row cell b blank t a n fraction numerator pi over denominator left square bracket x plus 4 right square bracket end fraction text end text text ( end text text x end text greater or equal than 0 text ) end text text end text end cell end table close text is continuous at end text x equals 0 text then end text$ the order pair (a,b)=

If $blank f blank open square brackets x close square brackets$ denotes the greatestinterger not exceeding x and if the function f defined by $blank f open parentheses x close parentheses equals open curly brackets table row cell fraction numerator a plus 2 blank c o s blank x over denominator x to the power of 2 end exponent end fraction blank left parenthesis x less than 0 right parenthesis end cell row cell b blank t a n fraction numerator pi over denominator left square bracket x plus 4 right square bracket end fraction text end text text ( end text text x end text greater or equal than 0 text ) end text text end text end cell end table close text is continuous at end text x equals 0 text then end text$ the order pair (a,b)=

$L e t blank a comma b blank element of R comma blank open parentheses a not equal to 0 close parentheses. blank I f blank t h e blank f u n c t i o n blank f blank d e f i n e d blank a s blank f open parentheses x close parentheses equals blank open curly brackets table row cell fraction numerator 2 x to the power of 2 end exponent over denominator a end fraction end cell cell 0 less or equal than x less than 1 end cell row a cell 1 less or equal than x less than square root of 2 end cell row cell fraction numerator 2 b to the power of 2 end exponent minus 4 b over denominator x to the power of 3 end exponent end fraction end cell cell square root of 2 less or equal than x less than 8 end cell end table close blank$ is continuous in the interval [0, $straight infinity$ ) then an ordered pair (a, b) is

$L e t blank a comma b blank element of R comma blank open parentheses a not equal to 0 close parentheses. blank I f blank t h e blank f u n c t i o n blank f blank d e f i n e d blank a s blank f open parentheses x close parentheses equals blank open curly brackets table row cell fraction numerator 2 x to the power of 2 end exponent over denominator a end fraction end cell cell 0 less or equal than x less than 1 end cell row a cell 1 less or equal than x less than square root of 2 end cell row cell fraction numerator 2 b to the power of 2 end exponent minus 4 b over denominator x to the power of 3 end exponent end fraction end cell cell square root of 2 less or equal than x less than 8 end cell end table close blank$ is continuous in the interval [0, $straight infinity$ ) then an ordered pair (a, b) is

parallel

Mosley measured the frequency (f) of the characteristic X-rays from many metals of different atomic number (Z) and represented his results by a relation known as Mosley's law. This law is (a, b are constants)

Mosley measured the frequency (f) of the characteristic X-rays from many metals of different atomic number (Z) and represented his results by a relation known as Mosley's law. This law is (a, b are constants)

Physics-General

X-rays which can penetrate through longer distances in substance are called

X-rays which can penetrate through longer distances in substance are called

Physics-General

X-rays are known to be electromagnetic radiations. Therefore the X-ray photon has

X-rays are known to be electromagnetic radiations. Therefore the X-ray photon has

Physics-General

parallel

When the accelerating voltage applied on the electrons increased beyond a critical value

When the accelerating voltage applied on the electrons increased beyond a critical value

Physics-General

X-rays are not used for radar purpose because

X-rays are not used for radar purpose because

Physics-General

X-rays were discovered by

X-rays were discovered by

Physics-General

parallel

In radio theraphy, X-rays are used to

In radio theraphy, X-rays are used to

Physics-General

The light rays having photons of energy 1.8 eV are falling on a metal surface having a work function 1.2 eV. What is the stopping potential to be applied to stop the emitting electrons

The light rays having photons of energy 1.8 eV are falling on a metal surface having a work function 1.2 eV. What is the stopping potential to be applied to stop the emitting electrons

Physics-General

The velocity of photon is proportional to (where n is frequency)

The velocity of photon is proportional to (where n is frequency)

Physics-General

parallel

card img

With Turito Academy.

card img

With Turito Foundation.

card img

Get an Expert Advice From Turito.

Turito Academy

card img

With Turito Academy.

Test Prep

card img

With Turito Foundation.