Have a query? Ask a Tutor!!

Access to best educators and exclusive paper discussions

Can’t find what you’re looking for?

Our tutors are from top schools around the world, and they are waiting for you 24/7, anytime, anywhere.

Homework- One to One Solutions
Understand concepts to solve better
Get your doubts solved instantly

Maths-

General

Easy

Question

An ellipse is drawn with major and minor axes of lengths 10 and 8 respectively. Using one focus as centre, a circle is drawn that is tangent to the ellipse, with no part of the circle being outside the ellipse. The radius of the circle is

$\sqrt{3}$
2
$2 \sqrt{2}$
$\sqrt{5}$

The correct answer is: 2

$table row cell 2 a equals 10 rightwards double arrow a equals 5 semicolon 2 b equals 8 rightwards double arrow b equals 4 end cell row cell c to the power of 2 end exponent equals 1 minus fraction numerator 16 over denominator 25 end fraction equals fraction numerator 9 over denominator 25 end fraction rightwards double arrow c equals fraction numerator 3 over denominator 5 end fraction end cell end table$

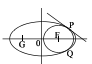
Focus = (3, 0)
Let the circle touches the ellipse at P and Q. Consider a tangent (to both circle and ellipse) at P. Let F(one focus) be the centre of the circle and other focus be G. A ray from F to P must retrace its path (normal to the circle). But the reflection propety the ray FP must be reflected along PG. This is possible only if P, F and G are collinear. Thus P must be the end of the major axis. Hence r = a – ae = 5 – 3 = 2 alternately normal to an ellipse at P must pass through the centre (3, 0) of the circle
$table row cell fraction numerator a x over denominator cos invisible function application theta end fraction minus fraction numerator b y over denominator sin invisible function application theta end fraction equals a to the power of 2 end exponent minus b to the power of 2 end exponent rightwards double arrow fraction numerator 5 x over denominator cos invisible function application theta end fraction minus fraction numerator 4 y over denominator sin invisible function application theta end fraction equals 9 open parentheses theta not equal to 0 text or end text fraction numerator pi over denominator 2 end fraction close parentheses end cell row cell fraction numerator 15 over denominator cos invisible function application theta end fraction minus 0 equals 9 rightwards double arrow c o s invisible function application theta equals fraction numerator 15 over denominator 9 end fraction rightwards double arrow text end text text w end text text h end text text i end text text c end text text h end text text end text text i end text text s end text text end text text n end text text o end text text t end text text end text text p end text text o end text text s end text text s end text text i end text text b end text text l end text text e end text text end text rightwards double arrow theta equals 0 text end text text o end text text r end text text end text pi divided by 2 end cell row cell text end text text b end text text u end text text t end text text end text theta not equal to pi divided by 2 rightwards double arrow theta equals 0 end cell row cell text end text text H end text text e end text text n end text text c end text text e end text text end text P equals left parenthesis 50 right parenthesis text end text text i end text text. end text text e end text text. end text text end text text e end text text n end text text d end text text end text text o end text text f end text text end text text m end text text a end text text j end text text o end text text r end text text end text text a end text text x end text text i end text text s end text text end text right square bracket end cell end table$

Related Questions to study

From a point P, perpendicular tangents PQ and PR are drawn to ellipse $x to the power of 2 end exponent plus 4 y to the power of 2 end exponent equals 4$ Locus of circumcentre of triangle PQR is

From a point P, perpendicular tangents PQ and PR are drawn to ellipse $x to the power of 2 end exponent plus 4 y to the power of 2 end exponent equals 4$ Locus of circumcentre of triangle PQR is

The least integral value that contained is the range of the function f defined by $f left parenthesis x right parenthesis equals fraction numerator x to the power of 2 end exponent plus 1 over denominator log invisible function application open parentheses x to the power of 2 end exponent plus 1 close parentheses end fraction$ is ___

The least integral value that contained is the range of the function f defined by $f left parenthesis x right parenthesis equals fraction numerator x to the power of 2 end exponent plus 1 over denominator log invisible function application open parentheses x to the power of 2 end exponent plus 1 close parentheses end fraction$ is ___

Let $f left parenthesis x right parenthesis equals L t stretchy sum from n rightwards arrow infinity to n minus 1 of fraction numerator x over denominator left parenthesis r x plus 1 right parenthesis left curly bracket left parenthesis r plus 1 right parenthesis x plus 1 right curly bracket end fraction comma$ then

Let $f left parenthesis x right parenthesis equals L t stretchy sum from n rightwards arrow infinity to n minus 1 of fraction numerator x over denominator left parenthesis r x plus 1 right parenthesis left curly bracket left parenthesis r plus 1 right parenthesis x plus 1 right curly bracket end fraction comma$ then

parallel

If m is the slope of tangent to the curve $e to the power of y end exponent equals 1 plus x to the power of 2 end exponent$ then

If m is the slope of tangent to the curve $e to the power of y end exponent equals 1 plus x to the power of 2 end exponent$ then

If $stack l i m with x rightwards arrow 0 below 1 plus x to the power of 2 end exponent plus fraction numerator f open parentheses x close parentheses over denominator x end fraction$ is equal to e⁷, then $stack l i m with x greater-than or slanted equal to 0 below fraction numerator f open parentheses x close parentheses over denominator x to the power of 3 end exponent end fraction equals horizontal ellipsis horizontal ellipsis$

If $stack l i m with x rightwards arrow 0 below 1 plus x to the power of 2 end exponent plus fraction numerator f open parentheses x close parentheses over denominator x end fraction$ is equal to e⁷, then $stack l i m with x greater-than or slanted equal to 0 below fraction numerator f open parentheses x close parentheses over denominator x to the power of 3 end exponent end fraction equals horizontal ellipsis horizontal ellipsis$

Set of all values of x such that $L t subscript x rightwards arrow infinity end subscript invisible function application fraction numerator 1 over denominator 1 plus open parentheses fraction numerator 4 t a n to the power of negative 1 end exponent invisible function application left parenthesis 2 pi x right parenthesis over denominator pi end fraction close parentheses to the power of 4 n end exponent end fraction$ is non-zero and finite number when n N $element of$ is

Set of all values of x such that $L t subscript x rightwards arrow infinity end subscript invisible function application fraction numerator 1 over denominator 1 plus open parentheses fraction numerator 4 t a n to the power of negative 1 end exponent invisible function application left parenthesis 2 pi x right parenthesis over denominator pi end fraction close parentheses to the power of 4 n end exponent end fraction$ is non-zero and finite number when n N $element of$ is

parallel

The period of the function $f left parenthesis x right parenthesis equals c o s e c to the power of 2 end exponent invisible function application 3 x plus c o t invisible function application 4 x$ is

The period of the function $f left parenthesis x right parenthesis equals c o s e c to the power of 2 end exponent invisible function application 3 x plus c o t invisible function application 4 x$ is

Let $g left parenthesis x right parenthesis equals left square bracket n plus p s i n invisible function application x right square bracket comma x element of left parenthesis 0 comma pi right parenthesis comma n element of Z comma p$ is a prime number and [x] = the greatest integer $less or equal than x$ The number of points at which f(x) is not differentiable is _______

Let $g left parenthesis x right parenthesis equals left square bracket n plus p s i n invisible function application x right square bracket comma x element of left parenthesis 0 comma pi right parenthesis comma n element of Z comma p$ is a prime number and [x] = the greatest integer $less or equal than x$ The number of points at which f(x) is not differentiable is _______

If the line $fraction numerator x minus 2 over denominator negative 1 end fraction equals fraction numerator y plus 2 over denominator 1 end fraction equals fraction numerator z plus k over denominator 4 end fraction$ is one of the angle bisectors of the lines $fraction numerator x over denominator 1 end fraction equals fraction numerator y over denominator negative 2 end fraction equals fraction numerator z over denominator 3 end fraction$ and $fraction numerator x over denominator negative 2 end fraction equals fraction numerator y over denominator 3 end fraction equals fraction numerator z over denominator 1 end fraction$ then the value of k is

If the line $fraction numerator x minus 2 over denominator negative 1 end fraction equals fraction numerator y plus 2 over denominator 1 end fraction equals fraction numerator z plus k over denominator 4 end fraction$ is one of the angle bisectors of the lines $fraction numerator x over denominator 1 end fraction equals fraction numerator y over denominator negative 2 end fraction equals fraction numerator z over denominator 3 end fraction$ and $fraction numerator x over denominator negative 2 end fraction equals fraction numerator y over denominator 3 end fraction equals fraction numerator z over denominator 1 end fraction$ then the value of k is

parallel

A particle when projected in vertical plane moves along smooth surface with initial velocity 20m/s at an angle of 60°, so that its normal reaction on the surface remains zero throughout the motion. Then the slope of the tangent to the surface at height 5 m from the point of projection will be

A particle when projected in vertical plane moves along smooth surface with initial velocity 20m/s at an angle of 60°, so that its normal reaction on the surface remains zero throughout the motion. Then the slope of the tangent to the surface at height 5 m from the point of projection will be

physics-General

Consider a particle moving without friction on a rippled surface, as shown. Gravity acts down in the negative h direction. The elevation h(x) of the surface is given by h(x) = d cos(kx). If the particle starts at x = 0 with a speed v in the x direction, for what values of v will the particle stay on the surface at all times?

Consider a particle moving without friction on a rippled surface, as shown. Gravity acts down in the negative h direction. The elevation h(x) of the surface is given by h(x) = d cos(kx). If the particle starts at x = 0 with a speed v in the x direction, for what values of v will the particle stay on the surface at all times?

physics-General

System shown in figure is released from rest. Pulley and spring is massless and friction is absent everywhere. The speed of 5 kg block when 2 kg block leaves the contact with ground is (Take force constant of spring $open k equals 40 N divided by m blank a n d blank g equals 10 m divided by s to the power of 2 end exponent close parentheses$

System shown in figure is released from rest. Pulley and spring is massless and friction is absent everywhere. The speed of 5 kg block when 2 kg block leaves the contact with ground is (Take force constant of spring $open k equals 40 N divided by m blank a n d blank g equals 10 m divided by s to the power of 2 end exponent close parentheses$

physics-General

parallel

A smooth track in the form of a quarter circle of radius 6 m lies in the vertical plane. A particle moves from $P subscript 1 end subscript blank t o blank P subscript 2 end subscript$ under the action of forces $stack F with rightwards arrow on top subscript 1 end subscript comma stack F with rightwards arrow on top subscript 2 end subscript blank a n d blank stack F with rightwards arrow on top subscript 3 end subscript. blank F o r c e blank stack F with rightwards arrow on top subscript 1 end subscript$ is always toward $P subscript 2 end subscript$ and is always 20 N in magnitude. Force $stack F with rightwards arrow on top subscript 2 end subscript$ always acts horizontally and is always 30 N in magnitude. Force $stack F with rightwards arrow on top subscript 3 end subscript$ always acts tangentially to the track and is of magnitude 15 N. Select the correct alternative(s)

A smooth track in the form of a quarter circle of radius 6 m lies in the vertical plane. A particle moves from $P subscript 1 end subscript blank t o blank P subscript 2 end subscript$ under the action of forces $stack F with rightwards arrow on top subscript 1 end subscript comma stack F with rightwards arrow on top subscript 2 end subscript blank a n d blank stack F with rightwards arrow on top subscript 3 end subscript. blank F o r c e blank stack F with rightwards arrow on top subscript 1 end subscript$ is always toward $P subscript 2 end subscript$ and is always 20 N in magnitude. Force $stack F with rightwards arrow on top subscript 2 end subscript$ always acts horizontally and is always 30 N in magnitude. Force $stack F with rightwards arrow on top subscript 3 end subscript$ always acts tangentially to the track and is of magnitude 15 N. Select the correct alternative(s)

physics-General

Ball I is thrown towards a tower at an angle of 60° with the horizontal with unknown speed (u). At the same moment ball II is released from the top of tower as shown. Balls collide after two seconds and at the moment of collision, velocity of ball I is horizontal. Find

Ball I is thrown towards a tower at an angle of 60° with the horizontal with unknown speed (u). At the same moment ball II is released from the top of tower as shown. Balls collide after two seconds and at the moment of collision, velocity of ball I is horizontal. Find

physics-General

A ball of mass ‘m’ is rotating in a circle of radius ‘r’ with speed v inside a smooth cone as shown in figure. Let N be the normal reaction on the ball by the cone, then choose the wrong option.

A ball of mass ‘m’ is rotating in a circle of radius ‘r’ with speed v inside a smooth cone as shown in figure. Let N be the normal reaction on the ball by the cone, then choose the wrong option.

physics-General

parallel

card img

With Turito Academy.

card img

With Turito Foundation.

card img

Get an Expert Advice From Turito.

Turito Academy

card img

With Turito Academy.

Test Prep

card img

With Turito Foundation.