The lines , m n are such that then

In the figure, ?

In the figure, p||q||r. Then ?

In the figure, and bisects

According to the figure, which of the following is true ?

In the figure, Find the value of x

In the given figure, p||q and r is transversal to p,q If

From the figure, Then ( x – y)

In the figure, if then

In the figure, n is transversal to lines then

In the figure, n is transversal to ,m then (x+y+z)-(p+q+r)=?

According to the data given in figure, x = ?

Ramesh and Mahesh solve an equation. In solving Ramesh commits a mistake in constant term and finds the roots 8 and 2. Mahesh commits a mistake in the coefficient of x and finds the roots – 9 and – 1. The correct roots are

Here we were given that in solving Ramesh commits a mistake in constant term and finds the roots 8 and 2. Many might create incorrect quadratic equations using the provided roots because they don't multiply carefully, which results in errors in one of the equation's signs. So the solution is 9, 1.

Three particles, each of mass m, are placed at the corners of right angled triangle as shown in Fig. If OA=a and OB=b, the position vector of the centre of mass is (here i and j are unit vectors along and axes respectively).

Four point masses and are placed on a plane as shown in figure. If the co-ordinates of the centre of mass of the system be then the values of and are respectively

Two blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on the first block ' A ' pulling it away from the other block 'B' as shown in figure at t=0. Extension of the spring is at time t.
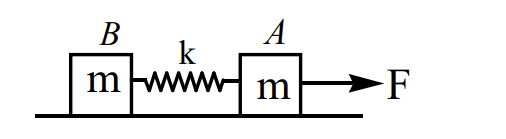Displacement of the centre of mass at time t is