Problems Involving Scale Drawing with Solved Examples

Key Concepts

• Scale drawing.
• Find actual lengths using scale drawings.
• Use scale factors to solve area problems.
• Reproduce a scale drawing at a different scale.

8.1 Solve problems involving scale drawings 

Scale drawing: 

A scale drawing is an enlarged or reduced drawing of an object that is proportional to the actual object. 

Solution: 

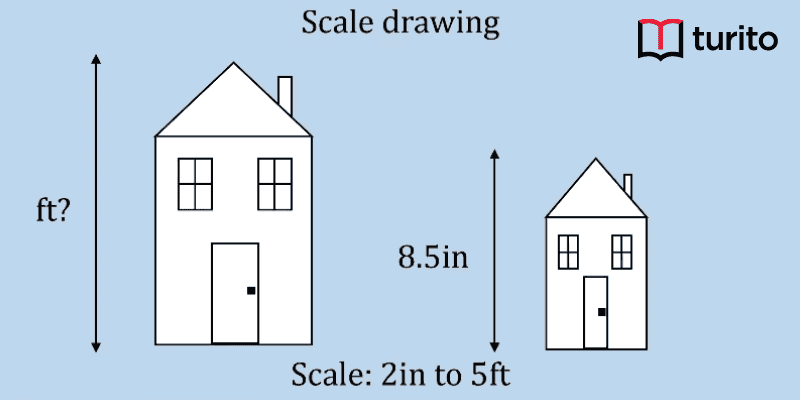
The image has two pictures of houses, the house on the right end is the reduced image of the actual house. Here, we are asked to find the height of the house on the left side. 

The scale here is mentioned as 2 inches = 5 feet. 

We are finding out the height of the actual house in feets, if it is 8.5 inches long. 

Use a proportion to find the actual length, x, of the island. 

5 / 2 = x / 8.5

Multiply by 8.5 on both sides of the equation. 

5 / 2 × 8.5 =

x / 8.5 x 8.5 =

42.5 / 2 = x 

x = 21.25 feet. 

Therefore, we conclude that the actual height of the house is 21.25 feet. 

8.1.1 Find actual lengths using scale drawing 

Example 1: Emily goes on a road trip. She is determining the distance she needs to cover using a map. The scale on her map reads 1 inch = 40 miles. She measures the distance to be 6 inches. Determine the distance of the trip. 

Solution: We observe that scale on the map is 1 inch = 40 miles. 

Step 1: Use a double number line to represent the scale drawing distance and the actual distance. 

Step 2: Use a proportion to find the actual distance, x of the trip. 

40/ 1 = x / 6

Multiply by 6 on both sides of the equation. 

40/1 × 6 = x6× 6 

40 × 6 / 1 = x 

x = 240 miles. 

Therefore, we conclude that Emily plans on 240-mile road trip. 

Example 2: The island in a blueprint is 2.5 inches in width. The scale used in the blueprint is 1 inch = 1.5 feet. What is width of the island? 

Solution: We observe that scale on the blueprint is 1 inch = 1.5 feet. 

Step 1: Use a double number line to represent the scale drawing width and the actual width. 

Step 2: Use a proportion to find the actual width, let ‘x’ be the width of the island. 

1.5 / 1 = x/ 2.5

Multiply by 2.5 on both sides of the equation. 

1.5 /1 × 2.5 = x / 2.5×2.5

1.5 × 2.5 / 1 = x 

x = 3.75 

Therefore, we conclude that width of the island is 3.75 feet. 

8.1.2 Use scale factors to solve area problems 

Example 1: An artist decides to paint below picture on the wall. He wants to enlarge the picture and uses the scale 1 cm = 5 yards to generalize the amount of area he needs to paint it. How much area should he need to do the painting? 

Solution: Use an equation in the form of y = kx to represent the proportional relationship between lengths in the scale drawing x, and actual lengths y. The constant of proportionality, k, is the scale factor. 

Step 1: Find the actual length L of the painting using the length l given. 

L = k × l 

L = 5 × 5 

L = 25 yards. 

Step 2: Find the actual width W of the painting using the width w given. 

W= k × w 

W = 5 × 5 

W = 25 yards. 

Step 3: Calculate the area of the painting on the wall 

Area = product of two adjacent sides 

Area = Length × width 

Area = 25 × 25 

Area = 625 square yards. 

Therefore, we conclude that area of the wall required to complete the painting is 625 square yards. 

Example 2: Determine the area, in square yards, of the garden in the shape of triangle, represented by the scale drawing.  

Solution: Use an equation in the form of y = kx to represent the proportional relationship between lengths in the scale drawing x, and actual lengths y. The constant of proportionality, k, is the scale factor. 

Step 1: Find the actual base B of the triangle using the base b given. 

B = k × b 

B = 2 × 4.5 

B = 9 yards. 

Step 2: Find the actual height H of the triangle using the height h given. 

H= k × h 

H= 2 × 6 

H = 12 yards. 

Step 3: Calculate the area of the garden in the shape of a triangle. 

Area of triangle =

1/2 × base × height 

Area of triangle =

1/2 × 9 × 12 

Area of triangle =

1 ×9 ×1/2 ×9 ×122

Area =108/2

Area = 54 square yards. 

Therefore, we conclude that area of garden in the shape of the triangle is 54 square yards. 

8.1.3 Reproduce a scale drawing at a different scale 

Example 1: The drawing below has a scale of 1 unit = 4 cm. Reproduce the drawing with a scale of 1 unit = 12 cm and calculate its area. 

Solution:  

Step 1: Find the actual length L using the length l in the scale drawing. 

Actual length L = k × x 

L = 9 × 4 

L = 36 centimetres. 

Step 2: Find the actual width W using the width in the scale drawing 

Actual width W = k × x 

W = 6 × 4 

W = 24 centimetres. 

Step 3: Find the new scale for the drawing. 

Take any dimension. In this case let us take length. 

36 cm ×1 unit / 12 cm

=36 units / 12

3 units. 

24 cm ×1 unit / 12 cm

=36 units / 12

2 units. 

Therefore, the reproduced drawing will have dimensions 3 units by 2 units. 

Example 2: The scale drawing shows represents an existing barn. They want the length of the barn to be 5 inches. What will be the new scale and the width of the barn? 

Solution:  

Step 1: Find the actual length L, and actual width W, of the barn. 

Determine the scale factor used to represent the drawing. 

Actual Length L = k × x 

L = 100 × 200 

L = 2000 meters. 

Actual width W = k × x 

W = 100 × 10 

W = 1000 meters. 

Step 2: Find the new scale for the barn to the actual barn. 

2000 meters / 5 inches = 400 meters / 1 inch

The new scale of 1 inch = 400 meters means 1 inch on the drawing is 400 meters of the actual barn. 

Step 3: Find the width using the new scale. 

W = k × w 

1000 = 400 × w 

1000 = 400 × w 

w = 2.5 inches. 

The dimensions of the new drawing will be 5 inches by 2.5 inches. 

Exercise:

1. Alexander draws a diagram of building on paper as 8 inches. If the scale used is 1 inch = 100 feet, determine the actual height of the building.
2. If the distance on the map represents 2 cm = 50 meters. What is the scale factor? 
3. On a map scale mentioned is 1 cm = 22 miles. If the distance between Hagerstown and Annapolis is shown as 6 cm on the map. Find the distance between the two places. 
4. What is the area in square feet, if a rhombus measuring 3 inch is represented by the scale drawing. The scale to be used is 1 inch = 3 feet. 
5. Determine the area of 10 cm by 8 cm playground shown in the blueprint, if 1 cm measures 24 feet. 
6. What is the area of square window, which has side measuring 0.75 inches and the scale used in drawing is 1 inch = 2 meters? 
7. 40 miles on a map is represented by a 5-inch line. If the map is enlarged to 3 times its size, what will be the scale of the enlarged map?  
8. The original 14-inch by 12-inch blueprint of a concrete patio has a scale of 2 inches = 3 feet. Adam wants to make a new blueprint of the patio with a length of 18 inches. What is the scale for the new blueprint? 
9. What is the actual base of the triangle?  

10. On a map 1 inch = 8 miles, two hotels are 8 inches apart. What is the distance between the two hotels?

What have we learned?

• What are scale drawings
• Finding actual lengths using scale drawings.
• Using scale factors to solve area problems.
• Reproduce a scale drawing at a different scale.