Maths-
General
Easy
Question
If  then
then 

We are given a function. It is function of two variables x and y. We should to find the value of fxy.
The correct answer is: 
The given function is f(x,y) = sin(eax + eby)
We have to find the value of fxy
It means we have to find the value of 
We will first take partial derivative w.r.t to one of the variable. Then we take the partial derivative of that value w.r.t the remaining variable.
Taking the partial derivative w.r.t x

We will take the derivative of this value w.r.t y

This is the required answer.
We have used rules of indices and different formulae of differentiation to solve the question. When we find the derivative w.r.t to some variable we take another variable as consta.
Related Questions to study
physics
The time period of a simple pendulum on a freely moving artificial satellite is
The time period of a simple pendulum on a freely moving artificial satellite is
physicsGeneral
physics
The radius of the earth is 6400 km and g= . In order that a body of 5 kg weights zero at the equator, the angular speed of the earth is =……rad/sec
. In order that a body of 5 kg weights zero at the equator, the angular speed of the earth is =……rad/sec
The radius of the earth is 6400 km and g= . In order that a body of 5 kg weights zero at the equator, the angular speed of the earth is =……rad/sec
. In order that a body of 5 kg weights zero at the equator, the angular speed of the earth is =……rad/sec
physicsGeneral
physics-
A transverse wave is represented by  . For what value of its wavelength will the wave velocity be equal to the maximum velocity of the particle taking part in the wave propagation?
. For what value of its wavelength will the wave velocity be equal to the maximum velocity of the particle taking part in the wave propagation?
A transverse wave is represented by  . For what value of its wavelength will the wave velocity be equal to the maximum velocity of the particle taking part in the wave propagation?
. For what value of its wavelength will the wave velocity be equal to the maximum velocity of the particle taking part in the wave propagation?
physics-General
physics
If R is the radius of the earth and g the acceleration due to gravity on the earth's surface, the mean density of the earth is =……
If R is the radius of the earth and g the acceleration due to gravity on the earth's surface, the mean density of the earth is =……
physicsGeneral
physics-
If. two almost identical waves having frequencies  and
and  produced one after the other superposes then the time interval to obtain a beat of maxirmum intensity is .......
produced one after the other superposes then the time interval to obtain a beat of maxirmum intensity is .......
If. two almost identical waves having frequencies  and
and  produced one after the other superposes then the time interval to obtain a beat of maxirmum intensity is .......
produced one after the other superposes then the time interval to obtain a beat of maxirmum intensity is .......
physics-General
physics-
As shown in figure, two pulses in a string having center to center distance of 8 cm are travelling abng mutually opposite direction. If the speed of both the pulse is  s, then after 2s, the energy of these pulses will be…………
s, then after 2s, the energy of these pulses will be…………

As shown in figure, two pulses in a string having center to center distance of 8 cm are travelling abng mutually opposite direction. If the speed of both the pulse is  s, then after 2s, the energy of these pulses will be…………
s, then after 2s, the energy of these pulses will be…………

physics-General
physics
As we go from the equator to the poles, the value of g…..
As we go from the equator to the poles, the value of g…..
physicsGeneral
physics
The gravitational force between two point masses  and
and  at separation r is given by F=
at separation r is given by F= The constant K…..
The constant K…..
The gravitational force between two point masses  and
and  at separation r is given by F=
at separation r is given by F= The constant K…..
The constant K…..
physicsGeneral
physics
Two point masses A and B having masses in the ratio 4:3 are seprated by a distance of 1m. When another point mass c of mass M is placed in between A and B the forces A and C is 1/3rd of the force between b and C, Then the distance C form is...m
Two point masses A and B having masses in the ratio 4:3 are seprated by a distance of 1m. When another point mass c of mass M is placed in between A and B the forces A and C is 1/3rd of the force between b and C, Then the distance C form is...m
physicsGeneral
physics
Which of the following statement about the gravitational constant is true
Which of the following statement about the gravitational constant is true
physicsGeneral
physics
Three equal masses of mkg each are plced the vertices of an equilateral triangle PQR and a mass of 2 m kg is placed at the centroid 0 of the triangle which is at a distance of m from each of vertices of triangle. The force in newton. acting on the mass 2 m is …….
Three equal masses of mkg each are plced the vertices of an equilateral triangle PQR and a mass of 2 m kg is placed at the centroid 0 of the triangle which is at a distance of m from each of vertices of triangle. The force in newton. acting on the mass 2 m is …….
physicsGeneral
biology
Match the column
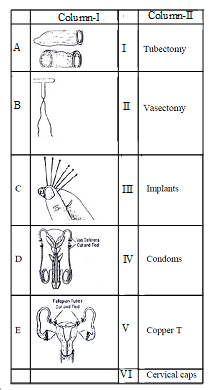
Match the column

biologyGeneral
physics-
As shown in figure, a block A having mass M is attached to one end of a massless spring. The block is on a frictionless horizontal surface and the free end of the spring is attached to a wall. Another block B having mass '  ' is placed on top of blockA. Now on displacing this system horizontally and released, it executes S.H.M. What should be the maximum amplitude of oscillation so that B does not slide off A? Coefficient of static friction between the surfaces of the block's is
' is placed on top of blockA. Now on displacing this system horizontally and released, it executes S.H.M. What should be the maximum amplitude of oscillation so that B does not slide off A? Coefficient of static friction between the surfaces of the block's is  .
.

As shown in figure, a block A having mass M is attached to one end of a massless spring. The block is on a frictionless horizontal surface and the free end of the spring is attached to a wall. Another block B having mass '  ' is placed on top of blockA. Now on displacing this system horizontally and released, it executes S.H.M. What should be the maximum amplitude of oscillation so that B does not slide off A? Coefficient of static friction between the surfaces of the block's is
' is placed on top of blockA. Now on displacing this system horizontally and released, it executes S.H.M. What should be the maximum amplitude of oscillation so that B does not slide off A? Coefficient of static friction between the surfaces of the block's is  .
.

physics-General
physics-
The displacement of a particle is given by 
 Which of the following graph represents variation in potential energy as a fimction of tims
Which of the following graph represents variation in potential energy as a fimction of tims  and displacement
and displacement  .
.


The displacement of a particle is given by 
 Which of the following graph represents variation in potential energy as a fimction of tims
Which of the following graph represents variation in potential energy as a fimction of tims  and displacement
and displacement  .
.


physics-General
physics-
Two blocks  and
and  are attached to the two ends of a spring having leagth
are attached to the two ends of a spring having leagth  and force consiant
and force consiant  on a horizontal surface. Initially the systen is in equilhium. Now a third block having same mass
on a horizontal surface. Initially the systen is in equilhium. Now a third block having same mass  , moving with velocity
, moving with velocity  collides with block A. Ln this situation........
collides with block A. Ln this situation........

Two blocks  and
and  are attached to the two ends of a spring having leagth
are attached to the two ends of a spring having leagth  and force consiant
and force consiant  on a horizontal surface. Initially the systen is in equilhium. Now a third block having same mass
on a horizontal surface. Initially the systen is in equilhium. Now a third block having same mass  , moving with velocity
, moving with velocity  collides with block A. Ln this situation........
collides with block A. Ln this situation........

physics-General



