Question
The probability of an unfair coin landing heads side up is 0.6. A student tossed this coin into the air 9 times. It landed tails side up 5 times and heads side up 4 times. What is the probability that the coin will land heads side up on the 10th toss?
- 0.4
- 0.5
- 0.6
- 1
The correct answer is: 0.6
HINT: Tossing of a coin is an independent event.
Complete step by step Solution
Since tossing of a coin is an independent event, the previous flips won't affect the forthcoming flips.
Given that the probability of an unfair coin landing heads side up = 0.6.
Since it is an independent event, the probability that the coin will land heads side up on the 10th toss is also 0.6.
Hence option C is the correct answer.
When a coin is flipped, it has unequal chances of landing heads-up or tails-up. • A Bernoulli trial is a random experiment with two possible outcomes, which are commonly referred to as success and failure, or the numeric values 1 and 0.
A fair coin has one tail and one head. Both of these outcomes have an equal chance of being the event's final outcome. For an unfair coin, the probability of seeing heads is 2/2 or 1. Similarly, the likelihood of a tail on an unfair coin is 2/2 or 1.
When a coin does not behave like a standard coin, it is said to be an Unfair Coin. Unfair Coin does not have the same outputs as a regular coin, for example, an Unfair Coin has either two heads or two tails.
Related Questions to study
The function 
is defined by 
. What is the value of f(3) ?
The function is defined by . What is the value of f(3) ?
The combustion of glucose releases energy. The ratio of grams of glucose combusted to kilocalories of energy released is 12.0 to 45.0. How many grams of glucose must be combusted to provide 85.5 kilocalories of energy?
The combustion of glucose releases energy. The ratio of grams of glucose combusted to kilocalories of energy released is 12.0 to 45.0. How many grams of glucose must be combusted to provide 85.5 kilocalories of energy?
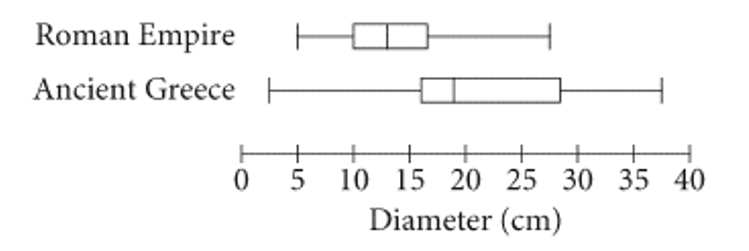
The Metropolitan Museum of Art has plates on display from the Roman Empire and ancient Greece. The box plots shown summarize the distributions of the diameters, in centimeters, of all the museum's plates from each region. How does the median diameter of the plates from the Roman Empire, r, compare to the median diameter of the plates from ancient Greece, g ?
The Metropolitan Museum of Art has plates on display from the Roman Empire and ancient Greece. The box plots shown summarize the distributions of the diameters, in centimeters, of all the museum's plates from each region. How does the median diameter of the plates from the Roman Empire, r, compare to the median diameter of the plates from ancient Greece, g ?
In 2015 , the city of Miami had a population of 441,000 people and an area of 36 square miles. What was the population density of Miami, in people per square mile, in 2015?
In 2015 , the city of Miami had a population of 441,000 people and an area of 36 square miles. What was the population density of Miami, in people per square mile, in 2015?
A sample of water was taken from each of ten different locations in a pond. The pH of each sample was measured. The measurements are summarized in the frequency table shown.
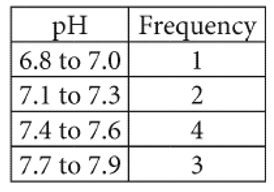
How many samples have a pH of 7.4 or greater?
A sample of water was taken from each of ten different locations in a pond. The pH of each sample was measured. The measurements are summarized in the frequency table shown.
How many samples have a pH of 7.4 or greater?
The function 
models the number of water hyacinths in a population over time, where A(t) is the number of water hyacinths and 
is the time, in days since the population was measured. Which is the best interpretation of 
in this context?
The function models the number of water hyacinths in a population over time, where A(t) is the number of water hyacinths and is the time, in days since the population was measured. Which is the best interpretation of in this context?
The graph of the function f is shown. Which of the following is the value of x for which f(x) = 0 ?
The graph of the function f is shown. Which of the following is the value of x for which f(x) = 0 ?
What is the graph of the equation 
?
What is the graph of the equation ?
Two beach balls are each in the shape of a sphere. The larger beach ball has a diameter of 3x, and the smaller beach ball has a diameter of x. What is the ratio of the volume of the larger beach ball to volume of the smaller beach ball?
volume of a sphere
A sphere is a collection of points in space separated by r from the center. The quantity of space a solid in three dimensions takes up is known as its volume. The unit of volume is measured in cubic (in3, ft3, cm3, m3, et cetera). Before calculating the volume, ensure all measurements are in the same unit.
Follow the steps below to determine the volume of a given sphere:
Step 1: Compare the radius of the given sphere. If you know the diameter of the sphere, divide it by two to get the radius.
Step 2: Find the radius of r'³s cube.
Step 3: Now divide it by (4/3) π
Step 4: The volume of the sphere will be the final answer.
The volume V of a sphere is equal to four-thirds of pi times the cube of the radius.
V = 4/3πr³
A hemisphere's volume is equal to one-half that of its related sphere.
Two beach balls are each in the shape of a sphere. The larger beach ball has a diameter of 3x, and the smaller beach ball has a diameter of x. What is the ratio of the volume of the larger beach ball to volume of the smaller beach ball?
volume of a sphere
A sphere is a collection of points in space separated by r from the center. The quantity of space a solid in three dimensions takes up is known as its volume. The unit of volume is measured in cubic (in3, ft3, cm3, m3, et cetera). Before calculating the volume, ensure all measurements are in the same unit.
Follow the steps below to determine the volume of a given sphere:
Step 1: Compare the radius of the given sphere. If you know the diameter of the sphere, divide it by two to get the radius.
Step 2: Find the radius of r'³s cube.
Step 3: Now divide it by (4/3) π
Step 4: The volume of the sphere will be the final answer.
The volume V of a sphere is equal to four-thirds of pi times the cube of the radius.
V = 4/3πr³
A hemisphere's volume is equal to one-half that of its related sphere.
?
, which of the following is equivalent to 
?
