An element, X has the following isotopic composition, 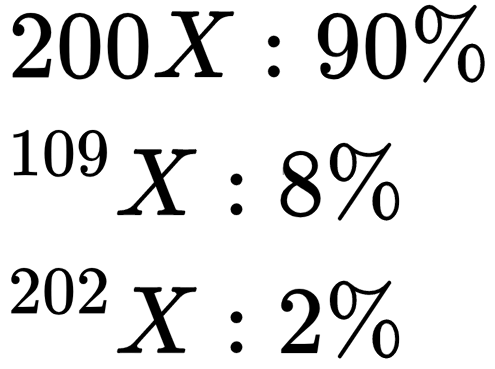the weighted average atomic mass of the naturally- occurring element 'X' is closest to :

The line x + my + n = 0 will touch the parabola y² = 4ax, if

a quadratic equation has equal roots if the D value is zero. this is the condition of the line touching the curve.

The straight line 2x + y –1= 0 meets the parabola y² = 4x in

when D> 0, two real and distinct roots
when D=0, real and equal roots
when D<0 imaginary roots.

The line y = mx + c may touch the parabola y² = 4a (x + a), if-

the solution of the two curves gives us the quadratic equation. D=0 gives us the required answer.

The straight line x + y = k touches the parabola y = x – x², if k =

since only one point of contact is present, D=0  gives the real root.

If the line x + y –1 = 0 touches the parabola y² = kx, then the value of k is-

we can also solve for the two lines and make the D=0 for the resultant quadratic equation.

If length of the two segments of focal chord to the parabola y² = 8ax are 2 and 4, then the value of a is-

the harmonic mean of the lengths (PS,QS) where S is the focus is equal to the half of latus rectum
the parametric coordinates of the ends of focal chords are (at^2, 2at) and (a/t^2,-2a/t)

If PSQ is the focal chord of the parabola y² = 8x such that SP = 6. Then the length SQ is-

the harmonic mean of the lengths (PS,QS) where S is the focus is equal to the half of latus rectum.

Length of the chord intercepted by the parabola y = x² + 3x  on the line x + y = 5 is

the distance between any two points is given by
√(x2-x1)²+(y2-y1)² 
this formula is applied to the points of intersection which were calculated by solving the two equations .

The length of the intercept made by the parabola 2y² + 6y = 8 – 5x on y-axis is

the distance between two points on the y axis is just the difference in the y coordinates of the points.

The length of the intercept made by the parabola x² –7x +4y +12= 0 on x-axis is

distance between two points on the x axis is just the difference between the x coordinates of the two points, since y =0.

The bond order is not three for -

The common features among the species CN^–,CO and NO⁺ are

How many σ and  π bond s will be in allyisoyanide:

Considering the state of hybridization of carbon atoms, find out the molecules among the following which is linear ?

Which of the following two are is o structural-