Maths-
SAT
Easy
Question
5x2 − 3(1 − x) − 2x(x + 5)
Which of the following polynomials is equivalent to the expression above?
- 3x2 − 7x − 3
- 3x2 + 7x − 3
- 5x2 − 5x − 3
- 5x2 − 9x − 3
The correct answer is: 3x2 − 7x − 3
5x2 – 3(1 - x) – 2x(x + 5)
5x2 – 3 + 3x – 2x2 – 10x
3x2 – 7x – 3
Related Questions to study
maths-
C = 10x + 4y
The formula above gives the monthly cost C, in dollars, of operating a delivery truck when the driver works a total of x hours and when y gallons of gasoline are used. If, in a particular month, it cost no more than $2,000 to operate the truck and at least 150 gallons of gas were used, what is the maximum number of hours the river could have worked?
C = 10x + 4y
The formula above gives the monthly cost C, in dollars, of operating a delivery truck when the driver works a total of x hours and when y gallons of gasoline are used. If, in a particular month, it cost no more than $2,000 to operate the truck and at least 150 gallons of gas were used, what is the maximum number of hours the river could have worked?
maths-sat
maths-
A farmer sold 108 pounds of produce that consisted of z pounds f zucchini and c pounds of cucumbers. The farmer sold the zucchini for $1.69 per pound and the cucumbers for $0.99 per pound and collected a total of $150.32. Which of the following systems of equations can be used to find the number of pounds of zucchini that were sold?
A farmer sold 108 pounds of produce that consisted of z pounds f zucchini and c pounds of cucumbers. The farmer sold the zucchini for $1.69 per pound and the cucumbers for $0.99 per pound and collected a total of $150.32. Which of the following systems of equations can be used to find the number of pounds of zucchini that were sold?
maths-sat
maths-
An instrument shows the number of revolutions per minute made by each tire of a car. In each revolution, the car travels a distance equal to the circumference of one of its tires. The circumference of each tire is equal to 2πr, where r is the radius of the tire.
Maria gets new tires for her car. The radius of each of her old tires is 0.30 meter, and the radius of each of her new tires is 11% larger than the radius of one of her old tires. What is the circumference of each new tire, to the nearest tenth of a meter?
An instrument shows the number of revolutions per minute made by each tire of a car. In each revolution, the car travels a distance equal to the circumference of one of its tires. The circumference of each tire is equal to 2πr, where r is the radius of the tire.
Maria gets new tires for her car. The radius of each of her old tires is 0.30 meter, and the radius of each of her new tires is 11% larger than the radius of one of her old tires. What is the circumference of each new tire, to the nearest tenth of a meter?
maths-sat
maths-
An instrument shows the number of revolutions per minute made by each tire of a car. In each revolution, the car travels a distance equal to the circumference of one of its tires. The circumference of each tire is equal to 2πr, where r is the radius of the tire.
If the radius of each tire on Maria’s car is 0.30 meter, what is the approximate speed of Maria’s car, to the nearest kilometer per hour, when the instrument is showing 779 revolutions per minute?
(1 kilometer = 1000 meters)
An instrument shows the number of revolutions per minute made by each tire of a car. In each revolution, the car travels a distance equal to the circumference of one of its tires. The circumference of each tire is equal to 2πr, where r is the radius of the tire.
If the radius of each tire on Maria’s car is 0.30 meter, what is the approximate speed of Maria’s car, to the nearest kilometer per hour, when the instrument is showing 779 revolutions per minute?
(1 kilometer = 1000 meters)
maths-sat
maths-
Ticket Prices by Row Number
|
Row number |
Ticket price |
|
1-2 |
$ 25 |
|
3-10 |
$20 |
|
11-20 |
$ 15 |
They will purchase 3 tickets for seats in row 1.
They will purchase 2 tickets for seats in row 3.
They will purchase 2 tickets for seats in row 4.
They will purchase 3 tickets for seats in row 12.
What is the average (arithmetic mean) ticket price, in dollars, for the 10 tickets? (Disregard the $ sign when gridding your answer.
Ticket Prices by Row Number
|
Row number |
Ticket price |
|
1-2 |
$ 25 |
|
3-10 |
$20 |
|
11-20 |
$ 15 |
They will purchase 3 tickets for seats in row 1.
They will purchase 2 tickets for seats in row 3.
They will purchase 2 tickets for seats in row 4.
They will purchase 3 tickets for seats in row 12.
What is the average (arithmetic mean) ticket price, in dollars, for the 10 tickets? (Disregard the $ sign when gridding your answer.
maths-sat
maths-
Century and Region of United States Presidents’ Births as of 2014
|
|
Century |
||
|
18th |
19th |
20th |
|
|
Northeast |
5 |
6 |
3 |
|
South |
9 |
4 |
3 |
|
Midwest |
0 |
9 |
2 |
|
West |
0 |
0 |
2 |
Century and Region of United States Presidents’ Births as of 2014
|
|
Century |
||
|
18th |
19th |
20th |
|
|
Northeast |
5 |
6 |
3 |
|
South |
9 |
4 |
3 |
|
Midwest |
0 |
9 |
2 |
|
West |
0 |
0 |
2 |
maths-sat
maths-
In the xy-plane, the graph of y = (x - 6)2 + 3 is the image of the graph of y = (x + 5)2 + 3 after a translation of how many units to the right?
In the xy-plane, the graph of y = (x - 6)2 + 3 is the image of the graph of y = (x + 5)2 + 3 after a translation of how many units to the right?
maths-sat
maths-
Which of the following equations describes a circle with radius 10 that passes through the origin when graphed in the xy-plane?
Which of the following equations describes a circle with radius 10 that passes through the origin when graphed in the xy-plane?
maths-sat
maths-
A researcher is investigating the relationship between the price of a four-pack of AA batteries at a convenience store and the number of competitors the store has. She defines a store’s competitor as another similar store within a 1-mile radius of the store she selects. She selects 32 convenience stores across a state at random, and for each store, she records the number of its competitors and its price for a four-pack of AA batteries. The results are shown, along with the line of best fit, in the scatterplot below.
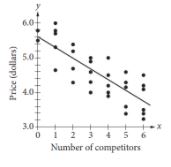
The line of best fit passes through the point (18, -0.12). Which of the following can be concluded from this?
A researcher is investigating the relationship between the price of a four-pack of AA batteries at a convenience store and the number of competitors the store has. She defines a store’s competitor as another similar store within a 1-mile radius of the store she selects. She selects 32 convenience stores across a state at random, and for each store, she records the number of its competitors and its price for a four-pack of AA batteries. The results are shown, along with the line of best fit, in the scatterplot below.

The line of best fit passes through the point (18, -0.12). Which of the following can be concluded from this?
maths-sat
maths-
A researcher is investigating the relationship between the price of a four-pack of AA batteries at a convenience store and the number of competitors the store has. She defines a store’s competitor as another similar store within a 1-mile radius of the store she selects. She selects 32 convenience stores across a state at random, and for each store, she records the number of its competitors and its price for a four-pack of AA batteries. The results are shown, along with the line of best fit, in the scatterplot below.
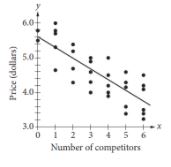
Another convenience store in the same state sells a four-pack of AA batteries for $4.89. If the store’s price is more than that predicted by the line of best fit, what is the least number of competitors the store could have?
A researcher is investigating the relationship between the price of a four-pack of AA batteries at a convenience store and the number of competitors the store has. She defines a store’s competitor as another similar store within a 1-mile radius of the store she selects. She selects 32 convenience stores across a state at random, and for each store, she records the number of its competitors and its price for a four-pack of AA batteries. The results are shown, along with the line of best fit, in the scatterplot below.

Another convenience store in the same state sells a four-pack of AA batteries for $4.89. If the store’s price is more than that predicted by the line of best fit, what is the least number of competitors the store could have?
maths-sat
maths-
2x + 3y = 5
4x + cy = 8
In the system of equations above, c is a constant. For what value of c will there be no solution (x, y) to the system of equations?
2x + 3y = 5
4x + cy = 8
In the system of equations above, c is a constant. For what value of c will there be no solution (x, y) to the system of equations?
maths-sat
maths-
The graphs in the xy-plane of the following quadratic equations each have x-intercepts of -2 and 4. The graph of which equation has its vertex farthest from the x-axis
The graphs in the xy-plane of the following quadratic equations each have x-intercepts of -2 and 4. The graph of which equation has its vertex farthest from the x-axis
maths-sat
maths-
On its opening day, a car dealership had an inventory of 29 cars. During the first 6 months, 18 additional cars were purchased by the dealership each week, and the sales team sold an average of 15 cars per week. During the first six months, which of the following equations best models the car inventory, c, at the dealership t weeks after opening day?
On its opening day, a car dealership had an inventory of 29 cars. During the first 6 months, 18 additional cars were purchased by the dealership each week, and the sales team sold an average of 15 cars per week. During the first six months, which of the following equations best models the car inventory, c, at the dealership t weeks after opening day?
maths-sat
maths-
Michael performed an experiment where he tossed two coins, one dime and one nickel, at the same time and recorded whether each one landed on heads or tails. His results are shown in the table below.
|
|
Nickel |
||
| Heads |
Tails |
||
| Dime |
Heads |
27 |
26 |
|
Tails |
24 |
23 |
|
Michael performed an experiment where he tossed two coins, one dime and one nickel, at the same time and recorded whether each one landed on heads or tails. His results are shown in the table below.
|
|
Nickel |
||
| Heads |
Tails |
||
| Dime |
Heads |
27 |
26 |
|
Tails |
24 |
23 |
|
maths-sat
maths-
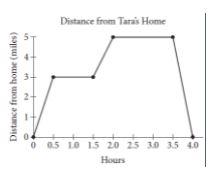
Tara rode her bicycle along a straight road from her home to a restaurant and ate lunch. She then continued along the same road to a movie theater to see a movie. Finally, she returned home on the same road after the movie. Tara’s distance from home during the 4 hours she was out is shown in the graph above. How many total miles did she ride her bicycle?

Tara rode her bicycle along a straight road from her home to a restaurant and ate lunch. She then continued along the same road to a movie theater to see a movie. Finally, she returned home on the same road after the movie. Tara’s distance from home during the 4 hours she was out is shown in the graph above. How many total miles did she ride her bicycle?
maths-sat





