Maths-
SAT
Easy
Question
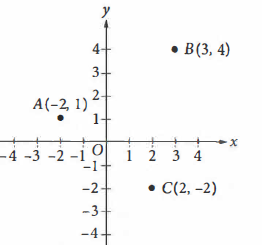
The coordinates of points A, B, and C are shown in the xy-plane above. For which of the following inequalities will each of the points A, B, and C be contained in the solution region?
- y > - x - 2
- y < x + 3
- x < 3
The correct answer is: y > - x - 2
Option A: y > – x – 2
A (-2,1) 1 > (-2) -2
1 > 0 True
B(3,4) 4 > -3 -2
4 > -5 True
C(2, -2) -2 > -2 -2
-2 > -4 True
Related Questions to study
Maths-
In the xy-plane, what is the y-coordinate of the y-intercept of the graph of the equation 
In the xy-plane, what is the y-coordinate of the y-intercept of the graph of the equation
Maths-SAT
Maths-
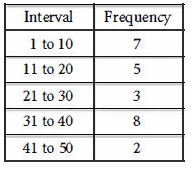
Each of the 25 data values in a data set is a different integer between 1 and 50, inclusive. The table gives the frequency of the data for five intervals. Which of the following intervals contains exactly 
of the values in the data set?
Each of the 25 data values in a data set is a different integer between 1 and 50, inclusive. The table gives the frequency of the data for five intervals. Which of the following intervals contains exactly of the values in the data set?
Maths-SAT
Maths-
In 1855, Louis Remme traveled from Sacramento, California, to Portland, Oregon, stopping to rest for only 10 hours of the 143 hours it took him to reach Portland. If his average speed while traveling without resting was 5 miles per hour, how many miles did Louis Remme travel?
In 1855, Louis Remme traveled from Sacramento, California, to Portland, Oregon, stopping to rest for only 10 hours of the 143 hours it took him to reach Portland. If his average speed while traveling without resting was 5 miles per hour, how many miles did Louis Remme travel?
Maths-SAT
Maths-
Based on the 2010 US census, the population of Milwaukee, Wisconsin, was about 96% of the population of Baltimore, Maryland. In 2010, if Milwaukee's population was about 595,000, which of the following is the best approximation of Baltimore's population?
Based on the 2010 US census, the population of Milwaukee, Wisconsin, was about 96% of the population of Baltimore, Maryland. In 2010, if Milwaukee's population was about 595,000, which of the following is the best approximation of Baltimore's population?
Maths-SAT
Maths-
A field has a perimeter of 960 feet. Of the following, which is closest to the perimeter of the field, in meters? (1 foot = 0.3048 meter)
A field has a perimeter of 960 feet. Of the following, which is closest to the perimeter of the field, in meters? (1 foot = 0.3048 meter)
Maths-SAT
Maths-
What is 120% of 2,000?
What is 120% of 2,000?
Maths-SAT
Maths-
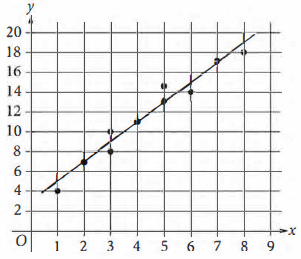
Ten data points are in the scatterplot shown, along with a line of best fit. Which of the following best estimates the predicted value of y when x = 6.5 ?
Ten data points are in the scatterplot shown, along with a line of best fit. Which of the following best estimates the predicted value of y when x = 6.5 ?
Maths-SAT
Maths-
If s = 4, what is the value of 20s - 15s ?
If s = 4, what is the value of 20s - 15s ?
Maths-SAT
Maths-
A square is inscribed in a circle with radius 6
inches. What is the perimeter of the square in inches?
A square's perimeter is the sum of the lengths of its four sides. As a result, we can calculate a square's perimeter by adding its four sides together. Each side of a square has the same length. Therefore, the side of a square is added four times to determine the perimeter of square.
Each side of a square with a 9-square-inch area is 3 inches long. Therefore, 3 inches multiplied by 3 inches equals 9 inches. (Length times height is a square's surface area formula.) A square has four sides; therefore, multiplying '3' by '4' gives us the perimeter, which is 12 inches.
A square is inscribed in a circle with radius 6 inches. What is the perimeter of the square in inches?
Maths-SAT
A square's perimeter is the sum of the lengths of its four sides. As a result, we can calculate a square's perimeter by adding its four sides together. Each side of a square has the same length. Therefore, the side of a square is added four times to determine the perimeter of square.
Each side of a square with a 9-square-inch area is 3 inches long. Therefore, 3 inches multiplied by 3 inches equals 9 inches. (Length times height is a square's surface area formula.) A square has four sides; therefore, multiplying '3' by '4' gives us the perimeter, which is 12 inches.
Maths-
3x - 0.6 = 1.8
What value of x satisfies the equation above?
3x - 0.6 = 1.8
What value of x satisfies the equation above?
Maths-SAT
Maths-
5(x - 3)(x + 1) = 0
What positive value of x satisfies the equation above?
5(x - 3)(x + 1) = 0
What positive value of x satisfies the equation above?
Maths-SAT
Maths-
x + y = 5
2x = 5
If (x, y) is the solution to the given system of equations, what is the value of y ?
x + y = 5
2x = 5
If (x, y) is the solution to the given system of equations, what is the value of y ?
Maths-SAT
Maths-
The graph of y = mx + b, where m and b are constants, is shown in the xy-plane.
What is the value of m?
The graph of y = mx + b, where m and b are constants, is shown in the xy-plane.
What is the value of m?
Maths-SAT
Maths-
When the speed of an object approaches the speed of light, its length as seen by an observer changes. When the object is stationary relative to an observer, its length is S, and when the same object is moving at speed v relative to the observer, its length is L. The formula above expresses L in terms of S, v, and c, the speed of light. Which of the following gives the speed of the object in terms of the other quantities?
When the speed of an object approaches the speed of light, its length as seen by an observer changes. When the object is stationary relative to an observer, its length is S, and when the same object is moving at speed v relative to the observer, its length is L. The formula above expresses L in terms of S, v, and c, the speed of light. Which of the following gives the speed of the object in terms of the other quantities?
Maths-SAT
Maths-
x2 -14x + 40 = 2x + 1
What is the sum of the solutions to the given equation?
x2 -14x + 40 = 2x + 1
What is the sum of the solutions to the given equation?
Maths-SAT
