Physics-
General
Easy
Question
A disc is rolling (without slipping) on a horizontal surface 
is its centre and 
and 
are two points equidistant from . Let 
, 
and 
be the magnitude of velocities of pints 
and respectively, then
,
,
The correct answer is:
In case of pure rolling bottommost point is the instantaneous centre of zero velocity.
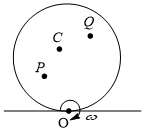
Velocity of any point on the disc, 
,
where 
is distance of point from 
.
Related Questions to study
Physics-
The acceleration of the center of mass of a uniform solid disc rolling down an inclined plane of angle 
is
The acceleration of the center of mass of a uniform solid disc rolling down an inclined plane of angle is
Physics-General
Physics-
A solid sphere of mass 
rolls down an inclined plane without slipping, starting from rest at the top of an inclined plane. The linear speed of the sphere at the bottom of the inclined plane is 
. The kinetic energy of the sphere at the bottom is
A solid sphere of mass rolls down an inclined plane without slipping, starting from rest at the top of an inclined plane. The linear speed of the sphere at the bottom of the inclined plane is . The kinetic energy of the sphere at the bottom is
Physics-General
Physics-
The moments of inertia of two freely rotating bodies 
and 
are 
and 
respectively. 
and their angular momenta are equal. If 
and 
are their kinetic energies, then
The moments of inertia of two freely rotating bodies and are and respectively. and their angular momenta are equal. If and are their kinetic energies, then
Physics-General
biology
Famous palaeonotologist/Palaeobotanist of India was:
Famous palaeonotologist/Palaeobotanist of India was:
biologyGeneral
physics-
A small object of uniform density roll up a curved surface with an initial velocity . If reaches up to a maximum height of 
with respect to the initial position. The object is
A small object of uniform density roll up a curved surface with an initial velocity . If reaches up to a maximum height of with respect to the initial position. The object is
physics-General
physics-
A uniform rod 
of length 
and mass is free to rotate about point . The rod is released from rest in the horizontal position. Given that the moment of inertia of the rod about is
, the initial angular acceleration of the rod will be
A uniform rod of length and mass is free to rotate about point . The rod is released from rest in the horizontal position. Given that the moment of inertia of the rod about is, the initial angular acceleration of the rod will be
physics-General
physics-
A particle of mass moves in the 
plane with a velocity along the straight line . If the angular momentum of the particle with respect to origin is 
when it is at and 
when it is at , then
A particle of mass moves in the plane with a velocity along the straight line . If the angular momentum of the particle with respect to origin is when it is at and when it is at , then
physics-General
maths-
Assertion (A): If the system of equations 
have non zero solution then 
Reason (R): If the system of equations 
has a non zero solution then 
is singular
Assertion (A): If the system of equations have non zero solution then
Reason (R): If the system of equations has a non zero solution then is singular
maths-General
physics-
Consider a body, shown in figure, consisting of two identical balls, each of mass 
connected by a light rigid rod. If an impulse
is impared to the body at one of its ends, what would be its angular velocity?
Consider a body, shown in figure, consisting of two identical balls, each of mass connected by a light rigid rod. If an impulse is impared to the body at one of its ends, what would be its angular velocity?
physics-General
physics-
A binary star consists of two stars (mass 2.2 
) and B (mass 11 ), where is the mass of the sun. They are separated by distance 
and are rotating about their centre of mass, which is stationary. The ratio of the total angular momentum of the binary star to the angular momentum of star about the centre of mass is
A binary star consists of two stars (mass 2.2 ) and B (mass 11 ), where is the mass of the sun. They are separated by distance and are rotating about their centre of mass, which is stationary. The ratio of the total angular momentum of the binary star to the angular momentum of star about the centre of mass is
physics-General
physics-
A force of- F 
acts on , the origin of the coordinate system. The torque about the point 1, -(a) is
A force of- F acts on , the origin of the coordinate system. The torque about the point 1, -(a) is
physics-General
Maths-
The area of the region bounded by y=|x-1| and y=1 in sq. units is
For such questions, we should know area of different shapes.
The area of the region bounded by y=|x-1| and y=1 in sq. units is
Maths-General
For such questions, we should know area of different shapes.
Maths-
The area of the elliptic quadratic with the semi major axis and semi minor axis as 6 and 4 respectively
For such questions, we should know the formula of area of different shapes.
The area of the elliptic quadratic with the semi major axis and semi minor axis as 6 and 4 respectively
Maths-General
For such questions, we should know the formula of area of different shapes.
physics-
A solid sphere of radius 
has moment of inertia 
about its geometrical axis. If it is melted into a disc of radius and thickness 
. If its moment of inertia about the tangential axis (which is perpendicular to plane of the disc), is also equal to , then the value of is equal to
A solid sphere of radius has moment of inertia about its geometrical axis. If it is melted into a disc of radius and thickness . If its moment of inertia about the tangential axis (which is perpendicular to plane of the disc), is also equal to , then the value of is equal to
physics-General
Maths-
The area of the region bounded by y=
and the x-axis is
The area of the region bounded by y= and the x-axis is
Maths-General
