Maths-
General
Easy
Question
The sides of a triangle are 
and 
for some 
.Then the greatest angle of the triangle is
- 600
- 1500
- 1200
- 900
Hint:
The angles of the triangle ABC are denoted by A, B, C and the corresponding opposite sides by a, b, c.
The correct answer is: 1200
The sides of a triangle are and for some .
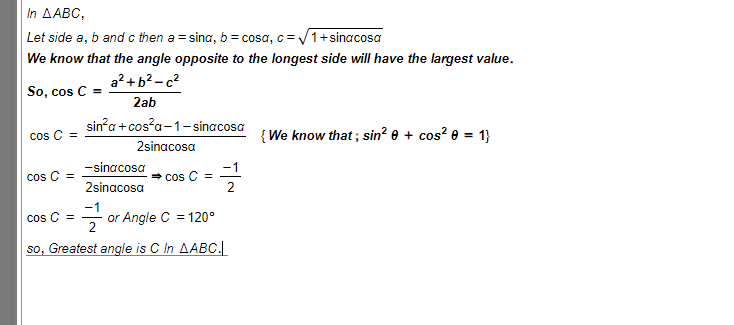
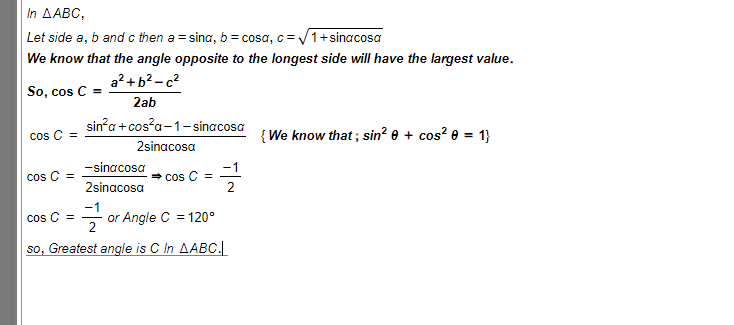
and for some .The law of cosines generalizes the Pythagorean formula to all triangles. It says that c2, the square of one side of the triangle, is equal to a2 + b2, the sum of the squares of the the other two sides, minus 2ab cos C, twice their product times the cosine of the opposite angle. When the angle C is right, it becomes the Pythagorean formula.
Related Questions to study
physics-
A cubical block of side a is moving with velocity V on a horizontal smooth plane as shown in figure. It hits a ridge at point O. The angular speed of the block after it hits 0 is
A cubical block of side a is moving with velocity V on a horizontal smooth plane as shown in figure. It hits a ridge at point O. The angular speed of the block after it hits 0 is
physics-General
physics-
A uniform rod of length 2 L is placed with one end in contact with horizontal and is then inclined at an angle 
to the horizontal and allowed to fall without slipping at contact point. When it becomes horizontal, its angular velocity will be.....
A uniform rod of length 2 L is placed with one end in contact with horizontal and is then inclined at an angle to the horizontal and allowed to fall without slipping at contact point. When it becomes horizontal, its angular velocity will be.....
physics-General
Maths-
If the sides of a triangle are in the ratio 
then greatest angle is
If the sides of a triangle are in the ratio then greatest angle is
Maths-General
physics-
A straight rod of length L has one of its ends at the origin and the other end at x=L If the mass per unit length of rod is given by Ax where A is constant where is its center of mass.
A straight rod of length L has one of its ends at the origin and the other end at x=L If the mass per unit length of rod is given by Ax where A is constant where is its center of mass.
physics-General
Maths-
In 
then 
s denotes the semi-perimeter of the triangle ABC, ∆ its area and R the radius of the circle circumscribing the triangle ABC i.e., R is the circum-radius.
In then
Maths-General
s denotes the semi-perimeter of the triangle ABC, ∆ its area and R the radius of the circle circumscribing the triangle ABC i.e., R is the circum-radius.
physics-
A small disc of radius 2 cm is cut from a disc of radius 6 cm. If the distance between their centrer is 3.2 cm, what is the shift in the center of mass of the disc...
A small disc of radius 2 cm is cut from a disc of radius 6 cm. If the distance between their centrer is 3.2 cm, what is the shift in the center of mass of the disc...
physics-General
physics-
Let be the moment of inertia of a uniform square plate about an axis AB that passes through its center and is parallel to two of its sides CD is a line in the plane of the plate that passes through the center of the plate and makes an angle of Q with AB. The moment of inertia of the plate about the axis CD is then equal to....
Let be the moment of inertia of a uniform square plate about an axis AB that passes through its center and is parallel to two of its sides CD is a line in the plane of the plate that passes through the center of the plate and makes an angle of Q with AB. The moment of inertia of the plate about the axis CD is then equal to....
physics-General
maths-
If 
is positive, then 
lies between
If is positive, then lies between
maths-General
Maths-
If 
then the Δk is
If then the Δk is
Maths-General
maths-
If 
then 
=
If then =
maths-General
maths-
If 
and 
, 
then 
If and , then
maths-General
maths-
In a 
If 
then 
In a If then
maths-General
maths-
Assertion (A): If 
then 
Reason (R): If 
then 
Assertion (A): If then
Reason (R): If then
maths-General
chemistry-
Concentrations of the atmospheric 
have been rising becaue of
Concentrations of the atmospheric have been rising becaue of
chemistry-General
physics-
Two disc of same thickness but of different radii are made of two different materials such that their masses are same. The densities of the materials are in the ratio 1:3. The moment of inertia of these disc about the respective axes passing through their center and perpendicular to their planes will be in the ratio
Two disc of same thickness but of different radii are made of two different materials such that their masses are same. The densities of the materials are in the ratio 1:3. The moment of inertia of these disc about the respective axes passing through their center and perpendicular to their planes will be in the ratio
physics-General
