Chemistry-
General
Easy
Question
The functional group present in acylchlorides is
- -Cl
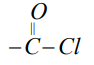

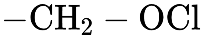
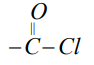
The correct answer is: 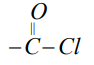
Related Questions to study
Maths-
If  are complex numbers such that
are complex numbers such that  are vertices of a triangle ABC,
are vertices of a triangle ABC,
 and
and  then
then
If  then the triangle is
then the triangle is
If  are complex numbers such that
are complex numbers such that  are vertices of a triangle ABC,
are vertices of a triangle ABC,
 and
and  then
then
If  then the triangle is
then the triangle is
Maths-General
Maths-
The general procedure for solving inequation containing modulus functions is to split the domain into subintervals and solve the various cases. But there are certain structures of equations which can be solved by a different approach.
For example:
i) The inequation  has no solution.
has no solution.
ii) Solving the inequaiton  is equivalent to solving the equations
is equivalent to solving the equations 
iii) Solving the inequation  is equivalent to solving the equations
is equivalent to solving the equations 
iv) Solving the inequation  is equivalent to solving the equations
is equivalent to solving the equations 
v) The inequation  is true for all
is true for all  domain of
domain of 
vi) The inequation  is true for all
is true for all  domain of
domain of 
Set of all real values of x for which  is defined, is
is defined, is
The general procedure for solving inequation containing modulus functions is to split the domain into subintervals and solve the various cases. But there are certain structures of equations which can be solved by a different approach.
For example:
i) The inequation  has no solution.
has no solution.
ii) Solving the inequaiton  is equivalent to solving the equations
is equivalent to solving the equations 
iii) Solving the inequation  is equivalent to solving the equations
is equivalent to solving the equations 
iv) Solving the inequation  is equivalent to solving the equations
is equivalent to solving the equations 
v) The inequation  is true for all
is true for all  domain of
domain of 
vi) The inequation  is true for all
is true for all  domain of
domain of 
Set of all real values of x for which  is defined, is
is defined, is
Maths-General
Maths-
Ten different letters of English alphabet are given. Words with five letters are formed from these given letters. Then, the number of words which have at least one letter repeated is
Ten different letters of English alphabet are given. Words with five letters are formed from these given letters. Then, the number of words which have at least one letter repeated is
Maths-General
Maths-
If two real valued functions f(x) & g(x) are given, for fog(x), g(x) is substituted in place of x in f(x) taking care of the domain of fog(x) which is subset of Domain of g(x), only those values of x such that g(x) lies in the Domain of f. Now, If  then g of (x) is
then g of (x) is
If two real valued functions f(x) & g(x) are given, for fog(x), g(x) is substituted in place of x in f(x) taking care of the domain of fog(x) which is subset of Domain of g(x), only those values of x such that g(x) lies in the Domain of f. Now, If  then g of (x) is
then g of (x) is
Maths-General
Maths-
where x, y, t R If t (5, k], then greatest value of k for which x is one-one function of t is
where x, y, t R If t (5, k], then greatest value of k for which x is one-one function of t is
Maths-General
Maths-
STATEMENT-I : If [4x] = [x], then the largest set of values of x is.  and
and
STATEMENT-II : If [nx] = [x],  then the largest set of values of x is
then the largest set of values of x is 
STATEMENT-I : If [4x] = [x], then the largest set of values of x is.  and
and
STATEMENT-II : If [nx] = [x],  then the largest set of values of x is
then the largest set of values of x is 
Maths-General
Maths-
 has
has
 has
has
Maths-General
Chemistry-
ClCH2COOH is heated with fuming HNO3 in the presence of AgNO3 in carius tube After filtration and washing a white precipitate is obtained The precipitate is of
ClCH2COOH is heated with fuming HNO3 in the presence of AgNO3 in carius tube After filtration and washing a white precipitate is obtained The precipitate is of
Chemistry-General
Chemistry-
Sodium extract gives blood red colour when treated with FeCl3 Formation of blood red colour confirms the presence of
Sodium extract gives blood red colour when treated with FeCl3 Formation of blood red colour confirms the presence of
Chemistry-General
Maths-
STATEMENT-I : The graph of consists of only two line segments. Where [.] represent greatest integer function and
consists of only two line segments. Where [.] represent greatest integer function and
STATEMENT-II : The graph of is
is

STATEMENT-I : The graph of consists of only two line segments. Where [.] represent greatest integer function and
consists of only two line segments. Where [.] represent greatest integer function and
STATEMENT-II : The graph of is
is

Maths-General
Maths-
Statement 1: Let be a continuous and onto function, then it cannot be one-one. And
be a continuous and onto function, then it cannot be one-one. And
Statement 2: If for a continuous and differentiable function f, then f(x) is one-one
then f(x) is one-one
Statement 1: Let be a continuous and onto function, then it cannot be one-one. And
be a continuous and onto function, then it cannot be one-one. And
Statement 2: If for a continuous and differentiable function f, then f(x) is one-one
then f(x) is one-one
Maths-General
Maths-
Number of solutions of the equation |3x 2| = 3[x] + 2{x}, where [.] and {.} denote the integral and fractional parts of x respectively, is
Number of solutions of the equation |3x 2| = 3[x] + 2{x}, where [.] and {.} denote the integral and fractional parts of x respectively, is
Maths-General
Chemistry-
A mixture of benzene and toluene can be separated by
A mixture of benzene and toluene can be separated by
Chemistry-General
Maths-
The solution set of the inequality log1/5 (2x + 5) + log5 (16 x 2) 1 is
The solution set of the inequality log1/5 (2x + 5) + log5 (16 x 2) 1 is
Maths-General
Maths-
Let f be an injective map with domain and range
and range such that exactly one of the following statements is correct and the remaining are false
such that exactly one of the following statements is correct and the remaining are false The value of
The value of is
is
For such questions, we should know concept of injective function.
Let f be an injective map with domain and range
and range such that exactly one of the following statements is correct and the remaining are false
such that exactly one of the following statements is correct and the remaining are false The value of
The value of is
is
Maths-General
For such questions, we should know concept of injective function.





