Question
Which statement below accurately represents the graph?

- $100 is earned for each hour.
- $100 is earned for every 2 hours.
- $500 is earned for every 8 hours.
- $200 is earned for every 3 hours.

Here we have given the graph of dollar earned vs hour worked. To match the statement read the graph carefully and find the coordinate for same point . Find the coordinate at x and y for same point. It Will help you to find the solution. Then match with the given options.
The correct answer is: $500 is earned for every 8 hours.
Here we have to find match which the statement is accurate.
Firstly , we have given graph, x-axis is minutes and y-axis is words.
According to graph, at x = 3 , we have y=200 ,that means
At 2 hour worked it has 200 dollar earned
So we can write,
3 hour worked = 200 dollar earned.

Therefore, $200 is earned for every 3 hours.
The correct answer is $200 is earned for every 3 hours (option (d)).
In this question, We have to find the dollar earned in hour and which statement is true. Just read the graph carefully find x and y coordinate for same point. Apply same concept in similar questions.
Related Questions to study
Identify the intervals in which the function is increasing.

For finding the increasing functions in interval you must consider the given interval will be going upward from left to right and you can see find the slope of interval if its positive then its increasing.
Identify the intervals in which the function is increasing.

For finding the increasing functions in interval you must consider the given interval will be going upward from left to right and you can see find the slope of interval if its positive then its increasing.
Identify the statement that best describes the increasing and decreasing of the graph.

It very easy to find the increasing and decreasing interval in graph you just have to focus on the graph where its going upward or downward or you can also find increasing and decreasing by finding the slope of the line. If the slope is positive then line is increasing otherwise slope is negative then line is decreasing.
Identify the statement that best describes the increasing and decreasing of the graph.

It very easy to find the increasing and decreasing interval in graph you just have to focus on the graph where its going upward or downward or you can also find increasing and decreasing by finding the slope of the line. If the slope is positive then line is increasing otherwise slope is negative then line is decreasing.
Which statement matches the graph?

In this question, we have to find the Taylor writing speed per minutes. Just read the graph carefully find x and y coordinate for same point, And then find y at x=1 . Apply same concept in similar questions.
Which statement matches the graph?

In this question, we have to find the Taylor writing speed per minutes. Just read the graph carefully find x and y coordinate for same point, And then find y at x=1 . Apply same concept in similar questions.
Find the constant rate of change.

Find the constant rate of change.

Identify whether the function is increasing, decreasing or constant at the interval pointed in the graph.

Identify whether the function is increasing, decreasing or constant at the interval pointed in the graph.

The table represents a linear function. What is the constant rate of change?

The table represents a linear function. What is the constant rate of change?

What is the definition of function?
Let us consider a function that defined as then, the representation may exist as shown below.
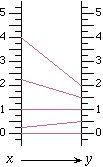
Hence, Every object x that belongs to the set of inputs has exactly one object y that belongs to the set of outputs.
*** Hence, the technical definition of the function is a relation from a set of i9nputs to set of possible outputs where each input is related to exactly one output.
What is the definition of function?
Let us consider a function that defined as then, the representation may exist as shown below.

Hence, Every object x that belongs to the set of inputs has exactly one object y that belongs to the set of outputs.
*** Hence, the technical definition of the function is a relation from a set of i9nputs to set of possible outputs where each input is related to exactly one output.



