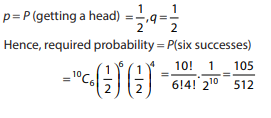Question
A coin is tossed 10 times The probability of getting exactly six heads is
- 512513
- 105512
- 100153
- ⌉0C6
The correct answer is: 105512
Related Questions to study
The letter of the word 'ASSASSlN' are written down at random in a row The probability that no two S occur together is
The letter of the word 'ASSASSlN' are written down at random in a row The probability that no two S occur together is
Let 

 be three mutually independent events Consider the Two Statement
be three mutually independent events Consider the Two Statement  and
and 
 :
:  and
and  are independent
are independent 
 and
and  are independent Then
are independent Then
Let 

 be three mutually independent events Consider the Two Statement
be three mutually independent events Consider the Two Statement  and
and 
 :
:  and
and  are independent
are independent 
 and
and  are independent Then
are independent Then
The locus of the foot of perpendicular drawn from the centre 
The locus of the foot of perpendicular drawn from the centre 
The equations of tangents to the ellipse  , which are inclined at 60° to x-axis, are
, which are inclined at 60° to x-axis, are
The equations of tangents to the ellipse  , which are inclined at 60° to x-axis, are
, which are inclined at 60° to x-axis, are
The area of the triangle formed by the lines joining the vertex of the parabola  to the ends of its latus rectum is
to the ends of its latus rectum is
The area of the triangle formed by the lines joining the vertex of the parabola  to the ends of its latus rectum is
to the ends of its latus rectum is
The length of chord of contact of the tangents drawn from the point (2, 5) to the parabola 
The length of chord of contact of the tangents drawn from the point (2, 5) to the parabola 
Area bounded by curve  ‐axis between
‐axis between  and
and  is
is
For such questions, we should know different formulas of integrals.
Area bounded by curve  ‐axis between
‐axis between  and
and  is
is
For such questions, we should know different formulas of integrals.
If  , then
, then  is equal to
is equal to
If  , then
, then  is equal to
is equal to
The integral  is equal to
is equal to
The integral  is equal to
is equal to
The solution for  of the equation
of the equation  is
is
The solution for  of the equation
of the equation  is
is
If the differential equation representing the family of all circles touching  ‐axis at the origin is
‐axis at the origin is  equals
equals
If the differential equation representing the family of all circles touching  ‐axis at the origin is
‐axis at the origin is  equals
equals
Which one of the following is a differential equation of the family of curves 
Which one of the following is a differential equation of the family of curves 
A function  has a second‐order derivatives
has a second‐order derivatives  1) If its graph passes through the point
1) If its graph passes through the point  and at that point the tangent to the grraph
and at that point the tangent to the grraph  , then the function is
, then the function is
For such questions, the important part is integration. We should know the methods to integrate the derivate. We should know the properties of a tangent.
A function  has a second‐order derivatives
has a second‐order derivatives  1) If its graph passes through the point
1) If its graph passes through the point  and at that point the tangent to the grraph
and at that point the tangent to the grraph  , then the function is
, then the function is
For such questions, the important part is integration. We should know the methods to integrate the derivate. We should know the properties of a tangent.
The differential equation  (
(  is any constant) represents
is any constant) represents
For such questions, we know different methods to solve differential equations. We should also know the formulas of different shapes.
The differential equation  (
(  is any constant) represents
is any constant) represents
For such questions, we know different methods to solve differential equations. We should also know the formulas of different shapes.