Question
A start-up company opened with 8 employees. The company’s growth plan assumes that 2 new employees will be hired each quarter (every 3 months) for the first 5 years. If an equation is written in the form y = ax + b to represent the number of employees, y, employed by the company x quarters after the company opened, what is the value of b ?
The correct answer is: 8
Given equation y = ax + b [ y = mx + c]
Here slope = a
Intercept = b
Since b is the intercept, and the intercept represents initial value at x = 0, thus b in the equation represents the initial number of employees.
Also, since the company started with 8 employees, b = 8
Related Questions to study
Volume 
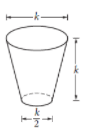
The glass pictured above can hold a maximum volume of 473 cubic centimeters, which is approximately 16 fluid ounces.
What is the value of k, in centimeters?
Volume 

The glass pictured above can hold a maximum volume of 473 cubic centimeters, which is approximately 16 fluid ounces.
What is the value of k, in centimeters?
Note: Figure not drawn to scale.
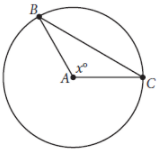
In the circle above, point A is the center and the length of arc  is
is  of the circumference of the circle. What is the value of x ?
of the circumference of the circle. What is the value of x ?

Note: Figure not drawn to scale.
In the circle above, point A is the center and the length of arc  is
is  of the circumference of the circle. What is the value of x ?
of the circumference of the circle. What is the value of x ?
−x + y = −3.5
x + 3y = 9.5
If (x, y) satisfies the system of equations above, what is the value of y ?
−x + y = −3.5
x + 3y = 9.5
If (x, y) satisfies the system of equations above, what is the value of y ?
The sum of −2x2 + x + 31 and 3x2 + 7x − 8 can be written in the form ax2 + bx + c, where a, b, and c are constants. What is the value of a + b + c ?
The sum of −2x2 + x + 31 and 3x2 + 7x − 8 can be written in the form ax2 + bx + c, where a, b, and c are constants. What is the value of a + b + c ?
x2 + x − 12 = 0
If a is a solution of the equation above and a > 0, what is the value of a ?
x2 + x − 12 = 0
If a is a solution of the equation above and a > 0, what is the value of a ?
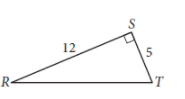
In triangle RST above, point W (not shown) lies on  . What is the value of cos(∠RSW) − sin(∠WST) ?
. What is the value of cos(∠RSW) − sin(∠WST) ?

In triangle RST above, point W (not shown) lies on  . What is the value of cos(∠RSW) − sin(∠WST) ?
. What is the value of cos(∠RSW) − sin(∠WST) ?

If x = 1 in the equation above, what is the value of a ?

If x = 1 in the equation above, what is the value of a ?
(x − 6)2 + (y + 5)2 = 16
In the xy-plane, the graph of the equation above is a circle. Point P is on the circle and has coordinates (10, −5). If  is a diameter of the circle, what are the coordinates of point Q ?
is a diameter of the circle, what are the coordinates of point Q ?
(x − 6)2 + (y + 5)2 = 16
In the xy-plane, the graph of the equation above is a circle. Point P is on the circle and has coordinates (10, −5). If  is a diameter of the circle, what are the coordinates of point Q ?
is a diameter of the circle, what are the coordinates of point Q ?
f (x) = x3 - 9x
g(x) = x2 - 2x - 3
Which of the following expressions is equivalent to  ?
?
f (x) = x3 - 9x
g(x) = x2 - 2x - 3
Which of the following expressions is equivalent to  ?
?
|
x |
g(x) |
|
-2 |
1 |
|
-1 |
2 |
|
0 |
3 |
|
1 |
4 |
|
2 |
5 |
|
3 |
6 |
|
4 |
7 |
|
x |
g(x) |
|
-2 |
1 |
|
-1 |
2 |
|
0 |
3 |
|
1 |
4 |
|
2 |
5 |
|
3 |
6 |
|
4 |
7 |
Volume 
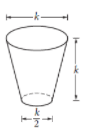
The glass pictured above can hold a maximum volume of 473 cubic centimeters, which is approximately 16 fluid ounces.
Jenny has a pitcher that contains 1 gallon of water. How many times could Jenny completely fill the glass with 1 gallon of water? (1 gallon = 128 fluid ounces)
Volume 

The glass pictured above can hold a maximum volume of 473 cubic centimeters, which is approximately 16 fluid ounces.
Jenny has a pitcher that contains 1 gallon of water. How many times could Jenny completely fill the glass with 1 gallon of water? (1 gallon = 128 fluid ounces)
In the xy-plane, the point (p, r) lies on the line with equation y = x + b, where b is a constant. The point with coordinates (2p, 5r) lies on the line with equation y = 2x + b. If p ≠ 0, what is the value of  ?
?
If we have coordinates of two points on a line, then we can use the slope formula to determine that line's slope. m = (y2-y1) / (x2-x1), or the ratio of the change in the y values and the x values, is the slope formula. 'x1' and 'y1' are the coordinates of the first point. The second points have the coordinates x2 and y2. If you have two points, you can always calculate the slope of a line. If you don't have access to two points, you can locate two points on the graph and use those to calculate the slope.
¶Observe the following helpful information:
- m = slope
¶-Rise = the difference between the y-values (y2 - y1), where (x1, y1) = point 1 and (x2, y2) = point 2.
Run is equal to the difference between x-values (x2 - x1).
In the xy-plane, the point (p, r) lies on the line with equation y = x + b, where b is a constant. The point with coordinates (2p, 5r) lies on the line with equation y = 2x + b. If p ≠ 0, what is the value of  ?
?
If we have coordinates of two points on a line, then we can use the slope formula to determine that line's slope. m = (y2-y1) / (x2-x1), or the ratio of the change in the y values and the x values, is the slope formula. 'x1' and 'y1' are the coordinates of the first point. The second points have the coordinates x2 and y2. If you have two points, you can always calculate the slope of a line. If you don't have access to two points, you can locate two points on the graph and use those to calculate the slope.
¶Observe the following helpful information:
- m = slope
¶-Rise = the difference between the y-values (y2 - y1), where (x1, y1) = point 1 and (x2, y2) = point 2.
Run is equal to the difference between x-values (x2 - x1).





