Which of the following expressions is equivalent to the given expression, where x -2?

(x³ + x) (x² -x)
Which of the following is equivalent to the given expression?

Aracely can spend up to a total of $20 on streamers and balloons for a party. Streamers cost $1.49 per pack. and balloons cost $4.39 per pack. Which of the following inequalities represents this situation, where s is the number of packs of streamers Aracely can buy, and b is the number of pack of balloons Aracely can buy? (Assume there is no sales tax.)

The function f is defined by f(x) = x² - 5x + 6. What is the value of f (4)?

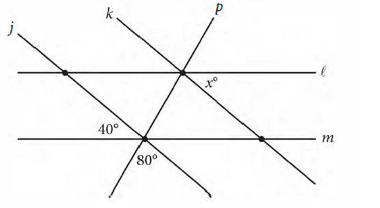
In the figure shown, line j is parallel to line k and line l is parallel to line m. What is the value of x ?

Interior angles are the angles contained within a shape or the region enclosed by two parallel lines transversely intersected.
¶Interior angles form in two ways in geometry. The first occurs within a polygon, and the second occurs when a transversal cuts parallel lines. Angles are classified into various types based on their measurements. Other angles are known as pair angles because they appear in pairs to exhibit a specific property. Interior angles are one example.
¶Sum, S = (n − 2) × 180°

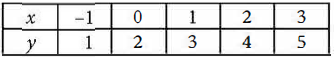
The table above shows some values of x and their corresponding values of y. Which of the following equations shows a possible relationship between x and y?

If , what is the value of

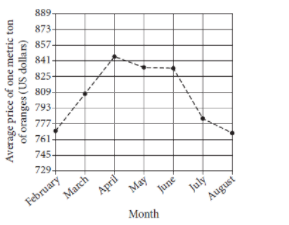
The line graph above shows the average price of one metric ton of oranges, in  dollars, for each of seven months in 2014.
Between which two consecutive months shown did the average price of one  metric ton of oranges decrease the most?

Use polynomial identities to factor the polynomials or simplify the expressions : 

Polynomial identities are equations that are true for all possible values of the variable. We can perform polynomial multiplication by applying the distributive property to the multiplication of polynomials.

Use the binomial theorem to expand the expressions: 

The answer can also be found using the Pascal’s triangle (using the fourth row).

Use the binomial theorem to expand the expressions: 

The answer can also be found using the Pascal’s triangle. For the expansion of the expression (x + y)n , we would consider the (n+1)th row in the triangle.

Use the binomial theorem to expand the expressions: 

The expansion of (x + y)n  can be also found using the Pascal’s triangle using the (n+1)th row of the triangle.

Use the binomial theorem to expand the expressions: 

The answer can also be found using the Pascal’s triangle. For the expansion of the expression (x + y)n  , we would consider the (n+1)th row in the triangle.

Use the binomial theorem to expand the expressions: 

The answer can also be found using the Pascal’s triangle. For the expansion of the expression (x + y)n, we would consider the (n+1)th row in the triangle.

Use the binomial theorem to expand the expressions: 

The answer can also be found using the Pascal’s triangle. For the expansion of the expression (x + y)n , we would consider the (n+1)th row in the triangle.

Use the binomial theorem to expand the expressions: 

The answer can also be found using the Pascal’s triangle. For the expansion of the expression (x + y)n , we would consider the (n+1)th row in the triangle.