Physics-
General
Easy
Question
A particle P is moving on a circle under the action of only one force acting always towards fixed point O on the circumference . The ratio of  and
and 


- 4 tan

- tan

- 2 tan

The correct answer is: 2 tan

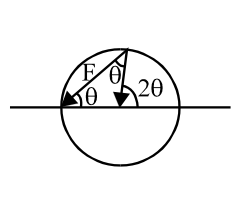
centripetal force = Fcosq = mw2r 

Þ tangential force = Fsinq



Ratio 
Related Questions to study
physics-
In the pulleys system as shown in the figure a particle of mass m falls from a height h0 on the mass m and gets stick to it. Speed with which the system moves just after the collision is

In the pulleys system as shown in the figure a particle of mass m falls from a height h0 on the mass m and gets stick to it. Speed with which the system moves just after the collision is

physics-General
physics-
A 10 kg block is pulled along a frictionless surface in the form of an arc of a circle of radius 10 m The applied force F is 200 N as shown in figure. If the block started from rest at P :

A 10 kg block is pulled along a frictionless surface in the form of an arc of a circle of radius 10 m The applied force F is 200 N as shown in figure. If the block started from rest at P :

physics-General
physics-
A square plate of mass m , length a is free to rotate about the axis XX¢ shown in figure . At a distance  from an edge . If is released from horizontal position shown in figure , find the angular speed attained by the plate , when it will pass through vertical position .
from an edge . If is released from horizontal position shown in figure , find the angular speed attained by the plate , when it will pass through vertical position .

A square plate of mass m , length a is free to rotate about the axis XX¢ shown in figure . At a distance  from an edge . If is released from horizontal position shown in figure , find the angular speed attained by the plate , when it will pass through vertical position .
from an edge . If is released from horizontal position shown in figure , find the angular speed attained by the plate , when it will pass through vertical position .

physics-General
physics-
A bob of mass m is suspended by a string from a train of mass M, free to move on a horizontal surface The bob is given a horizontal velocity V0 The maximum height attained by the bob is

A bob of mass m is suspended by a string from a train of mass M, free to move on a horizontal surface The bob is given a horizontal velocity V0 The maximum height attained by the bob is

physics-General
physics-
A small block is released from a point A of a rough semicircular track as shown in figure. When it moves along the track :

A small block is released from a point A of a rough semicircular track as shown in figure. When it moves along the track :

physics-General
physics-
A man standing on flat car under the shed of a small umbrella as shown in figure Rainfall starts at 30 m/s at an angle 30° with the vertical as shown. Find the speed and direction in which flatcar should be moved in order to save man from rain fall .

A man standing on flat car under the shed of a small umbrella as shown in figure Rainfall starts at 30 m/s at an angle 30° with the vertical as shown. Find the speed and direction in which flatcar should be moved in order to save man from rain fall .

physics-General
physics-
In the diagram shown, the rod is rigid and massless. A constant force F = mg has been applied vertically downwards on the rod The wall and the horizontal surface are perfectly smooth If the initial value of the angle  is equal to 45° then the minimum value of coefficient of friction between the blocks for on relative motion to start between the blocks, is equal to
is equal to 45° then the minimum value of coefficient of friction between the blocks for on relative motion to start between the blocks, is equal to

In the diagram shown, the rod is rigid and massless. A constant force F = mg has been applied vertically downwards on the rod The wall and the horizontal surface are perfectly smooth If the initial value of the angle  is equal to 45° then the minimum value of coefficient of friction between the blocks for on relative motion to start between the blocks, is equal to
is equal to 45° then the minimum value of coefficient of friction between the blocks for on relative motion to start between the blocks, is equal to

physics-General
physics-
A particle of mass m is projected at an angle of 60° with a velocity of 20 m/s relative to the ground from a plank of same mass m which is placed on smooth surface Initially plank was at rest The minimum length of the plank for which the ball will fall on the plank itself is

A particle of mass m is projected at an angle of 60° with a velocity of 20 m/s relative to the ground from a plank of same mass m which is placed on smooth surface Initially plank was at rest The minimum length of the plank for which the ball will fall on the plank itself is

physics-General
maths-
Statement‐I:: The values of a for which the point  lies inside the triangle formed by the lines x=0, x+y=2, and 3y=x is (0,1) .
lies inside the triangle formed by the lines x=0, x+y=2, and 3y=x is (0,1) .
Statement‐II:: The parabola  meets the line
meets the line  at (1, 1) .
at (1, 1) .
Statement‐I:: The values of a for which the point  lies inside the triangle formed by the lines x=0, x+y=2, and 3y=x is (0,1) .
lies inside the triangle formed by the lines x=0, x+y=2, and 3y=x is (0,1) .
Statement‐II:: The parabola  meets the line
meets the line  at (1, 1) .
at (1, 1) .
maths-General
Maths-
Tangents are drawn from the point (-1,2) on the parabola  The length, these tangents will intercept on the line
The length, these tangents will intercept on the line  :
:
Hence finally the length is
Tangents are drawn from the point (-1,2) on the parabola  The length, these tangents will intercept on the line
The length, these tangents will intercept on the line  :
:
Maths-General
Hence finally the length is
Maths-
Let A and B be two points on a parabola  with vertex V such that VA is perpendicular to VB and θ is the angle between the chord VA and the axis of the parabola The value of
with vertex V such that VA is perpendicular to VB and θ is the angle between the chord VA and the axis of the parabola The value of  is
is
Let A and B be two points on a parabola  with vertex V such that VA is perpendicular to VB and θ is the angle between the chord VA and the axis of the parabola The value of
with vertex V such that VA is perpendicular to VB and θ is the angle between the chord VA and the axis of the parabola The value of  is
is
Maths-General
maths-
The equation of the parabola whose vertex and focus lie on the axis of  at distances a and
at distances a and  from the origin, respectively, is
from the origin, respectively, is
The equation of the parabola whose vertex and focus lie on the axis of  at distances a and
at distances a and  from the origin, respectively, is
from the origin, respectively, is
maths-General
Maths-
Consider,  where p is a real number, and
where p is a real number, and 
Statement‐l:: If line  is a chord of circle
is a chord of circle  , then line
, then line  is not always adiameter ofcircle C.
is not always adiameter ofcircle C.
Statement‐II :: If line  is a diameter ofcircle
is a diameter ofcircle  , then line
, then line  is not a chord ofcircle C.
is not a chord ofcircle C.
Consider,  where p is a real number, and
where p is a real number, and 
Statement‐l:: If line  is a chord of circle
is a chord of circle  , then line
, then line  is not always adiameter ofcircle C.
is not always adiameter ofcircle C.
Statement‐II :: If line  is a diameter ofcircle
is a diameter ofcircle  , then line
, then line  is not a chord ofcircle C.
is not a chord ofcircle C.
Maths-General
Maths-
The equation of the circle passing through the points (1, 0) and (0,1) and having the smallest radius is:
Therefore the correct option is Choice 3
The equation of the circle passing through the points (1, 0) and (0,1) and having the smallest radius is:
Maths-General
Therefore the correct option is Choice 3
Maths-
If a circle passes through the point (a, b) and cuts the circle  orthogonally, then the equation of the locus of its centre is‐
orthogonally, then the equation of the locus of its centre is‐
Hence locus of the given condition is optionD
If a circle passes through the point (a, b) and cuts the circle  orthogonally, then the equation of the locus of its centre is‐
orthogonally, then the equation of the locus of its centre is‐
Maths-General
Hence locus of the given condition is optionD





