Question
John drained the water in his tub. The amount of water left in the tub (in liters) as a function of time (in minutes) is graphed.

Identify the input variable.
- Water
- Time
- Minutes
- Cannot say

John drained the water in his tub. In that given graph we can look x-axis as a time and y-axis as a water. We need to focus on graph whether that is given that the find out the input variable of graph.
The correct answer is: Time
Here we need to find the which is the input variable
Firstly, in a graph x-axis which is also horizontal axis is the time of graph.
And , y-axis is which is vertical axis is the Amount of water at a time t minutes
And we know that along the x-axis change in time comes to change in water.
At first water is 360 liter,
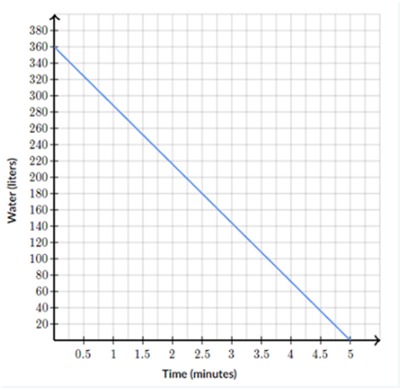
After 5 minutes its completely drained.
So in time vs water , time is input variable . Because change in time cause the draining of water
The correct answer is time (option (b)).
The input variable are change or measured along the horizontal axis or x-axis and the output variable along the vertical axis or y-axis.
Related Questions to study
How many increasing/decreasing intervals are there in this graph?

It very easy to find the increasing and decreasing interval in graph you just have to focus on the graph where its going upward or downward or you can also find increasing and decreasing by finding the slope of the line. If the slope is positive then line is increasing otherwise slope is negative then line is decreasing.
How many increasing/decreasing intervals are there in this graph?

It very easy to find the increasing and decreasing interval in graph you just have to focus on the graph where its going upward or downward or you can also find increasing and decreasing by finding the slope of the line. If the slope is positive then line is increasing otherwise slope is negative then line is decreasing.
What is the slope in the equation y = 2x + 3?
For finding the slope you need to remember the equation of the slope form of straight line is y=mx+b, where m is slope and b is intercept .
And also the slope is also called as tanƟ or m.
What is the slope in the equation y = 2x + 3?
For finding the slope you need to remember the equation of the slope form of straight line is y=mx+b, where m is slope and b is intercept .
And also the slope is also called as tanƟ or m.
What is the y-intercept of the following graph?

The y-intercept can be easily found by the locating the point of the line which cuts on y-axis and always remember the x-coordinate will be zero.
What is the y-intercept of the following graph?

The y-intercept can be easily found by the locating the point of the line which cuts on y-axis and always remember the x-coordinate will be zero.
John drained the water in his tub. The amount of water left in the tub (in liters) as a function of time (in minutes) is graphed.

Identify the out variable?
The input variable are change or measured along the horizontal axis or x-axis and the output variable along the vertical axis or y-axis.
John drained the water in his tub. The amount of water left in the tub (in liters) as a function of time (in minutes) is graphed.

Identify the out variable?
The input variable are change or measured along the horizontal axis or x-axis and the output variable along the vertical axis or y-axis.
Identify whether the function is increasing, decreasing or constant at the interval pointed in the graph.

For finding increasing, decreasing and constant interval in any particular interval you must look line whose going downward , upward or horizontal and also find by finding slope of interval .
Identify whether the function is increasing, decreasing or constant at the interval pointed in the graph.

For finding increasing, decreasing and constant interval in any particular interval you must look line whose going downward , upward or horizontal and also find by finding slope of interval .
Identify the intervals in which the function is decreasing.

For finding the decreasing functions in interval you must consider the given interval will be going downward from left to right .
Identify the intervals in which the function is decreasing.

For finding the decreasing functions in interval you must consider the given interval will be going downward from left to right .
Look at the graph below and identify whether the function at interval 2 is increasing, decreasing or constant.

You can apply can process throughout any question related to increasing, decreasing and constant interval where you can find out easily by looking the graph or slope of line.
Look at the graph below and identify whether the function at interval 2 is increasing, decreasing or constant.

You can apply can process throughout any question related to increasing, decreasing and constant interval where you can find out easily by looking the graph or slope of line.
Mike, who is a bicyclist is riding along a flat road. He then goes downhill. Can you identify the output variable when you represent the distance traveled over time with a qualitative graph?
The input variable are change or measured along the horizontal axis or x-axis and the output variable along the vertical axis or y-axis. And here distance on which is dependent variable because it is at y-axis.
Mike, who is a bicyclist is riding along a flat road. He then goes downhill. Can you identify the output variable when you represent the distance traveled over time with a qualitative graph?
The input variable are change or measured along the horizontal axis or x-axis and the output variable along the vertical axis or y-axis. And here distance on which is dependent variable because it is at y-axis.
Davin keeps a record in graph form of how far his car travels and the number of gallons of gasoline it uses. What is the number of gallons that Davin uses per 32 miles?

To find the solution we have focus on the given coordinate on the graph. It will help you to find the x and y coordinate by that you can easily find the solution. And also find for one thing first and then by that you can easily find given thing. Apply the same concept in similar questions.
Davin keeps a record in graph form of how far his car travels and the number of gallons of gasoline it uses. What is the number of gallons that Davin uses per 32 miles?

To find the solution we have focus on the given coordinate on the graph. It will help you to find the x and y coordinate by that you can easily find the solution. And also find for one thing first and then by that you can easily find given thing. Apply the same concept in similar questions.
How many increasing and decreasing intervals are there in this graph?

It very easy to find the increasing and decreasing interval in graph you just have to focus on the graph where its going upward or downward or you can also find increasing and decreasing by finding the slope of the line. If the slope is positive then line is increasing otherwise slope is negative then line is decreasing.
How many increasing and decreasing intervals are there in this graph?

It very easy to find the increasing and decreasing interval in graph you just have to focus on the graph where its going upward or downward or you can also find increasing and decreasing by finding the slope of the line. If the slope is positive then line is increasing otherwise slope is negative then line is decreasing.
Look at the graph below and identify whether the function at interval 1 is increasing, decreasing or constant.

You can apply can process throughout any question related to increasing, decreasing and constant interval where you can find out easily by looking the graph or slope of line.
Look at the graph below and identify whether the function at interval 1 is increasing, decreasing or constant.

You can apply can process throughout any question related to increasing, decreasing and constant interval where you can find out easily by looking the graph or slope of line.
Which statement below accurately represents the graph?

In this question, We have to find the dollar earned in hour and which statement is true. Just read the graph carefully find x and y coordinate for same point. Apply same concept in similar questions.
Which statement below accurately represents the graph?

In this question, We have to find the dollar earned in hour and which statement is true. Just read the graph carefully find x and y coordinate for same point. Apply same concept in similar questions.
Identify the intervals in which the function is increasing.

For finding the increasing functions in interval you must consider the given interval will be going upward from left to right and you can see find the slope of interval if its positive then its increasing.
Identify the intervals in which the function is increasing.

For finding the increasing functions in interval you must consider the given interval will be going upward from left to right and you can see find the slope of interval if its positive then its increasing.
Identify the statement that best describes the increasing and decreasing of the graph.

It very easy to find the increasing and decreasing interval in graph you just have to focus on the graph where its going upward or downward or you can also find increasing and decreasing by finding the slope of the line. If the slope is positive then line is increasing otherwise slope is negative then line is decreasing.
Identify the statement that best describes the increasing and decreasing of the graph.

It very easy to find the increasing and decreasing interval in graph you just have to focus on the graph where its going upward or downward or you can also find increasing and decreasing by finding the slope of the line. If the slope is positive then line is increasing otherwise slope is negative then line is decreasing.
Which statement matches the graph?

In this question, we have to find the Taylor writing speed per minutes. Just read the graph carefully find x and y coordinate for same point, And then find y at x=1 . Apply same concept in similar questions.
Which statement matches the graph?

In this question, we have to find the Taylor writing speed per minutes. Just read the graph carefully find x and y coordinate for same point, And then find y at x=1 . Apply same concept in similar questions.



