Question
In the figure, ‘x° ’ and ‘y° ’ are two exterior angle measures of 
. Then x° + y° is
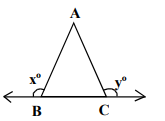
- 90°
- < 90°
- 180°
- > 180°
Hint:
sum of all exterior angles of a triangle = 360 degrees. sum of 2 obtuse angles is > 180 degrees.
The correct answer is: > 180°
>180
From the given diagram, we can see that angles x and y are obtuse angles, i.e., both are > 90 degree.
Therefore, their sum, x+ y > 90 + 90 or x + y > 180 degrees.
if one of the angles were acute then we'd not have been able to conclude whether the sum of the angles were greater than or less than 180 degrees.
Related Questions to study
The perimeter of the following figure is
perimeter is the sum of the sides of a polygon.
The perimeter of the following figure is
perimeter is the sum of the sides of a polygon.
Two pupils Ram and Shyam have taken lime water in a vessel, as shown in the figure. Ram is inhaling air from straw A and Shyam is blowing air through straw B. In which case lime water turns milky faster and Why?
Two pupils Ram and Shyam have taken lime water in a vessel, as shown in the figure. Ram is inhaling air from straw A and Shyam is blowing air through straw B. In which case lime water turns milky faster and Why?
If 
where 
then A is equal to
If where then A is equal to
The range of the function 
In this question, we have to find the range of f(x)=
. Here solve the function and find when function is at maximum and minimum.
The range of the function
In this question, we have to find the range of f(x)=. Here solve the function and find when function is at maximum and minimum.
The range of the function 
The range of the function
The domain of the function 
The domain of the function
If 
, and the period of 
is 
, then n is equal to
If , and the period of is , then n is equal to
Domain of definition of the function 
Domain of definition of the function
Range of the function 
Range of the function
Range of the function
Range of the function
The domain of 
The domain of
The domain of the function 
The domain of the function
If 
, then 
is
Differentiation is the process of determining a function's derivative. The derivative is the rate at which x changes in relation to y when x and y are two variables. A constant function has zero derivatives. For instance, f'(x) = 0 if f(x) = 8. So the derivative function is odd.
If , then is
Differentiation is the process of determining a function's derivative. The derivative is the rate at which x changes in relation to y when x and y are two variables. A constant function has zero derivatives. For instance, f'(x) = 0 if f(x) = 8. So the derivative function is odd.
is
