Have a query? Ask a Tutor!!

Access to best educators and exclusive paper discussions

Offsite Campus Turito Championship

Can’t find what you’re looking for?

Our tutors are from top schools around the world, and they are waiting for you 24/7, anytime, anywhere.

Homework- One to One Solutions
Understand concepts to solve better
Get your doubts solved instantly

Maths-

General

Easy

Question

Statement I : $blank to the power of 21 end exponent C subscript 0 end subscript plus blank to the power of 21 end exponent C subscript 1 end subscript plus horizontal ellipsis plus blank to the power of 21 end exponent C subscript 10 end subscript equals 2 to the power of 20 end exponent$ because
Statement II : $blank to the power of 2 n plus 1 end exponent C subscript 0 end subscript plus blank to the power of 2 n plus 1 end exponent C subscript 1 end subscript plus horizontal ellipsis to the power of 2 n plus 1 end exponent C subscript 2 n plus 1 end subscript equals 2 to the power of 2 n plus 1 end exponent$ and ⁿC_r = ⁿC_n_r

Statement 1 is True, Statement 2 is True; Statement 2 is a correct explanation for Statement 1
Statement 1 is True, Statement 2 is Tue; Statement 2 is NOT a correct explanation for Statement 1.
Statement 1 is True, Statement 2 is False
Statement 1 is False, statement2 is True.

The correct answer is: Statement 1 is True, Statement 2 is True; Statement 2 is a correct explanation for Statement 1

.

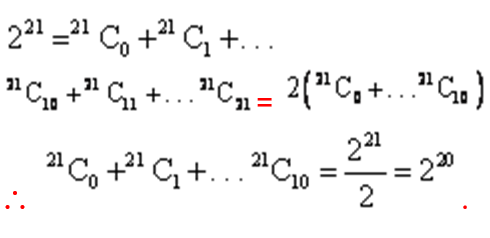Related Questions to study

If C_r denotes the combinatorial coefficient in the expansion of (1 + x)ⁿ then (nN
Statement 1: $left parenthesis n plus 1 right parenthesis open parentheses C subscript 0 end subscript superscript 2 end superscript plus C subscript 1 end subscript superscript 2 end superscript plus C subscript 2 end subscript superscript 2 end superscript plus horizontal ellipsis plus C subscript n end subscript superscript 2 end superscript close parentheses greater than open parentheses C subscript 0 end subscript plus C subscript 1 end subscript plus C subscript 2 end subscript plus horizontal ellipsis plus C subscript n end subscript close parentheses to the power of 2 end exponent for all n greater or equal than 2 comma n element of N$ because
Statement 2: Root mean square of n distinct real numbers is always greater than their arithmetic mean, n ≥ 2

If C_r denotes the combinatorial coefficient in the expansion of (1 + x)ⁿ then (nN
Statement 1: $left parenthesis n plus 1 right parenthesis open parentheses C subscript 0 end subscript superscript 2 end superscript plus C subscript 1 end subscript superscript 2 end superscript plus C subscript 2 end subscript superscript 2 end superscript plus horizontal ellipsis plus C subscript n end subscript superscript 2 end superscript close parentheses greater than open parentheses C subscript 0 end subscript plus C subscript 1 end subscript plus C subscript 2 end subscript plus horizontal ellipsis plus C subscript n end subscript close parentheses to the power of 2 end exponent for all n greater or equal than 2 comma n element of N$ because
Statement 2: Root mean square of n distinct real numbers is always greater than their arithmetic mean, n ≥ 2

The sum $stretchy sum from k equals 1 to n minus gamma plus 1 of k to the power of n minus k end exponent C subscript gamma minus 1 end subscript$ is equal to

The sum $stretchy sum from k equals 1 to n minus gamma plus 1 of k to the power of n minus k end exponent C subscript gamma minus 1 end subscript$ is equal to

$stretchy sum from i equals 0 to n of stretchy sum from j equals 0 to n of stretchy sum from k equals 0 to n of open parentheses fraction numerator n over denominator i end fraction close parentheses open parentheses fraction numerator n over denominator k end fraction close parentheses comma open parentheses fraction numerator n over denominator r end fraction close parentheses equals blank to the power of n end exponent C subscript r end subscript$

$stretchy sum from i equals 0 to n of stretchy sum from j equals 0 to n of stretchy sum from k equals 0 to n of open parentheses fraction numerator n over denominator i end fraction close parentheses open parentheses fraction numerator n over denominator k end fraction close parentheses comma open parentheses fraction numerator n over denominator r end fraction close parentheses equals blank to the power of n end exponent C subscript r end subscript$

parallel

The expression $open parentheses table row cell n end cell row cell k end cell end table close parentheses plus open parentheses table row cell n end cell row cell k plus 1 end cell end table close parentheses$ where $n greater or equal than k greater or equal than 1$ is the same as

The expression $open parentheses table row cell n end cell row cell k end cell end table close parentheses plus open parentheses table row cell n end cell row cell k plus 1 end cell end table close parentheses$ where $n greater or equal than k greater or equal than 1$ is the same as

Which of the following pairs of ions cannot beseparated by H₂Sindilute HCl?

Which of the following pairs of ions cannot beseparated by H₂Sindilute HCl?

Chemistry-General

The pairofcom pounds which cannot exist together in solution is:

The pairofcom pounds which cannot exist together in solution is:

Chemistry-General

parallel

If the greatest value of the term independent of x in expansion of $open parentheses x s i n invisible function application p plus x to the power of negative 1 end exponent c o s invisible function application p close parentheses to the power of 10 end exponent$ is achieved at $P equals theta$ Then the locus of point from which pair of tangents be drawn to $x to the power of 2 end exponent plus y to the power of 2 end exponent equals 4$ including an angle $theta$ is

If the greatest value of the term independent of x in expansion of $open parentheses x s i n invisible function application p plus x to the power of negative 1 end exponent c o s invisible function application p close parentheses to the power of 10 end exponent$ is achieved at $P equals theta$ Then the locus of point from which pair of tangents be drawn to $x to the power of 2 end exponent plus y to the power of 2 end exponent equals 4$ including an angle $theta$ is

The sum of the series $fraction numerator 5 over denominator 1.4 to the power of 2 end exponent end fraction plus fraction numerator 11 over denominator 4 to the power of 2 end exponent times 7 to the power of 2 end exponent end fraction plus fraction numerator 17 over denominator 7 to the power of 2 end exponent times 10 to the power of 2 end exponent end fraction plus horizontal ellipsis.. plus$ is equal to

The sum of the series $fraction numerator 5 over denominator 1.4 to the power of 2 end exponent end fraction plus fraction numerator 11 over denominator 4 to the power of 2 end exponent times 7 to the power of 2 end exponent end fraction plus fraction numerator 17 over denominator 7 to the power of 2 end exponent times 10 to the power of 2 end exponent end fraction plus horizontal ellipsis.. plus$ is equal to

In the expansion of $open parentheses x plus x to the power of 2 end exponent plus horizontal ellipsis horizontal ellipsis.. close parentheses open parentheses 1 plus x plus x to the power of 2 end exponent plus x to the power of 3 end exponent close parentheses open parentheses x to the power of 2 end exponent plus horizontal ellipsis horizontal ellipsis. plus x to the power of 10 end exponent close parentheses$ the coefficient of is

In the expansion of $open parentheses x plus x to the power of 2 end exponent plus horizontal ellipsis horizontal ellipsis.. close parentheses open parentheses 1 plus x plus x to the power of 2 end exponent plus x to the power of 3 end exponent close parentheses open parentheses x to the power of 2 end exponent plus horizontal ellipsis horizontal ellipsis. plus x to the power of 10 end exponent close parentheses$ the coefficient of is

Maths-General

parallel

Statement 1:The coefficient of $x to the power of n end exponent$ in the binomial expansion of $left parenthesis 1 minus x right parenthesis to the power of negative 2 end exponent$ is $left parenthesis n plus 1 right parenthesis$
Statement 2:The coefficient of $x to the power of r end exponent$ in $left parenthesis 1 minus x right parenthesis to the power of negative n end exponent$ when $n element of N$ is $blank to the power of n plus r minus 1 end exponent C subscript r end subscript$

Statement 1:The coefficient of $x to the power of n end exponent$ in the binomial expansion of $left parenthesis 1 minus x right parenthesis to the power of negative 2 end exponent$ is $left parenthesis n plus 1 right parenthesis$
Statement 2:The coefficient of $x to the power of r end exponent$ in $left parenthesis 1 minus x right parenthesis to the power of negative n end exponent$ when $n element of N$ is $blank to the power of n plus r minus 1 end exponent C subscript r end subscript$

If the $left parenthesis r plus 1 right parenthesis$ th term in the expansion of $open parentheses fraction numerator a to the power of 1 divided by 3 end exponent over denominator b to the power of 1 divided by 6 end exponent end fraction plus fraction numerator b to the power of 1 divided by 2 end exponent over denominator a to the power of 1 divided by 6 end exponent end fraction close parentheses to the power of 21 end exponent$ has equal exponents of both $a$ and $b$ , then value of $r$ is

If the $left parenthesis r plus 1 right parenthesis$ th term in the expansion of $open parentheses fraction numerator a to the power of 1 divided by 3 end exponent over denominator b to the power of 1 divided by 6 end exponent end fraction plus fraction numerator b to the power of 1 divided by 2 end exponent over denominator a to the power of 1 divided by 6 end exponent end fraction close parentheses to the power of 21 end exponent$ has equal exponents of both $a$ and $b$ , then value of $r$ is

If in the expansion of $left parenthesis 1 plus x right parenthesis to the power of n end exponent$ , the coefficient of $r$ th and $left parenthesis r plus 2 right parenthesis$ th term be equal, then $r$ is equal to

If in the expansion of $left parenthesis 1 plus x right parenthesis to the power of n end exponent$ , the coefficient of $r$ th and $left parenthesis r plus 2 right parenthesis$ th term be equal, then $r$ is equal to

parallel

The sum of the series $not stretchy sum from r equals 0 to n of left parenthesis negative 1 right parenthesis to the power of r end exponent blank to the power of n end exponent C subscript r end subscript open parentheses fraction numerator 1 over denominator 2 to the power of r end exponent end fraction plus fraction numerator 3 to the power of r end exponent over denominator 2 to the power of 2 r end exponent end fraction plus fraction numerator 7 to the power of r end exponent over denominator 2 to the power of 3 r end exponent end fraction plus fraction numerator 15 to the power of r end exponent over denominator 2 to the power of 4 r end exponent end fraction plus... plus m blank t e r m s close parentheses$ is

The sum of the series $not stretchy sum from r equals 0 to n of left parenthesis negative 1 right parenthesis to the power of r end exponent blank to the power of n end exponent C subscript r end subscript open parentheses fraction numerator 1 over denominator 2 to the power of r end exponent end fraction plus fraction numerator 3 to the power of r end exponent over denominator 2 to the power of 2 r end exponent end fraction plus fraction numerator 7 to the power of r end exponent over denominator 2 to the power of 3 r end exponent end fraction plus fraction numerator 15 to the power of r end exponent over denominator 2 to the power of 4 r end exponent end fraction plus... plus m blank t e r m s close parentheses$ is

The coefficient of $t to the power of 24 end exponent$ in the expansion of $open parentheses 1 plus t to the power of 2 end exponent close parentheses to the power of 12 end exponent open parentheses 1 plus t to the power of 12 end exponent close parentheses left parenthesis 1 plus t to the power of 24 end exponent right parenthesis$ is

The coefficient of $t to the power of 24 end exponent$ in the expansion of $open parentheses 1 plus t to the power of 2 end exponent close parentheses to the power of 12 end exponent open parentheses 1 plus t to the power of 12 end exponent close parentheses left parenthesis 1 plus t to the power of 24 end exponent right parenthesis$ is

The coefficient of $x to the power of 5 end exponent$ in the expansion of $open parentheses 1 plus x to the power of 2 end exponent close parentheses to the power of 5 end exponent open parentheses 1 plus x close parentheses to the power of 4 end exponent$ is

The coefficient of $x to the power of 5 end exponent$ in the expansion of $open parentheses 1 plus x to the power of 2 end exponent close parentheses to the power of 5 end exponent open parentheses 1 plus x close parentheses to the power of 4 end exponent$ is

parallel

card img

With Turito Academy.

card img

With Turito Foundation.

card img

Get an Expert Advice From Turito.

Turito Academy

card img

With Turito Academy.

Test Prep

card img

With Turito Foundation.