Question
The relative yield of corn, expressed as a percent, and soil pH were recorded for 5 samples. For the 5 samples, the relative yield of corn reached a maximum when the soil had a  of
of  , and then decreased as the soil pH increased. Which scatterplot could represent these data?
, and then decreased as the soil pH increased. Which scatterplot could represent these data?

The correct answer is: 
Observation of graph will be performed to solve the question.
Explanations:
Step 1 of 1:

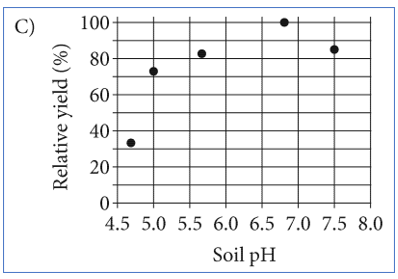
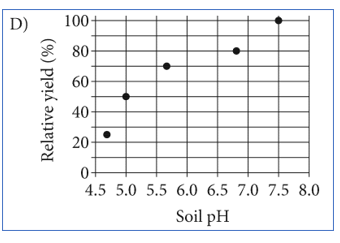
Looking at the above options of the graphs, we can observe that
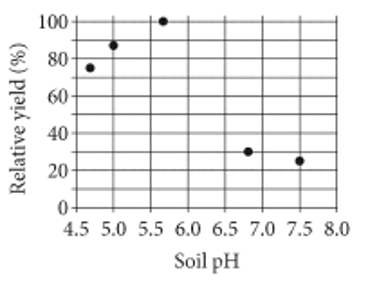
For A, at 6.8 soil pH, the relative yield (%) is 30 and then at pH 7.5, yield is decreased to 25.
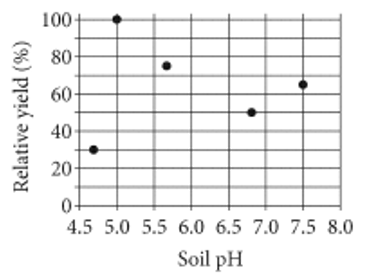
For B, at 6.8 soil pH, the relative yield (%) is 50 and then at pH 7.5, yield is increased to 65.
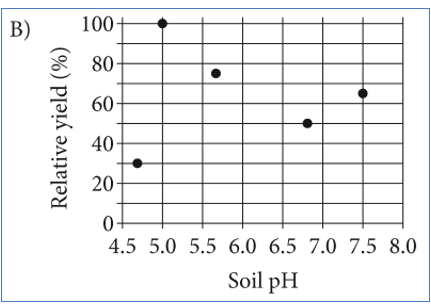
For C, at 6.8 soil pH, the relative yield (%) is 100 and then at pH 7.5, yield is decreased to 85.
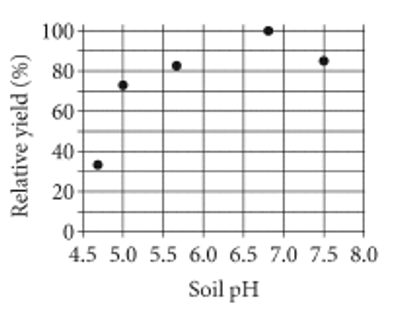
For D, at 6.8 soil pH, the relative yield (%) is 80 and then at pH  , yield is increased to 100.
, yield is increased to 100.
So, according to the question, option C graph has the maximum relative yield of corn when the soil had a pH of  and then decreased as the soil pH increased.
and then decreased as the soil pH increased.
Final Answer:
The scatterplot—
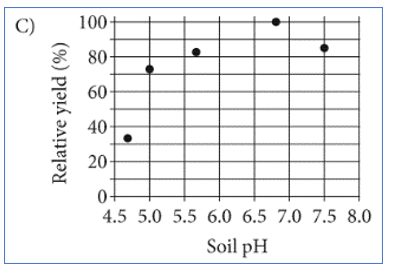 would represent these data.
would represent these data.
Related Questions to study
The table shows the maximum depth, in meters, of the 5 deepest oceanic trenches.
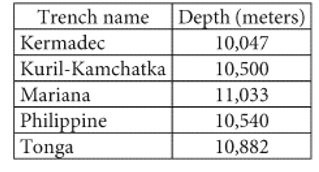
What is the range, in meters, of these 5 trench depths?
The table shows the maximum depth, in meters, of the 5 deepest oceanic trenches.

What is the range, in meters, of these 5 trench depths?
Each of the whole numbers from 1 to 20 is written on one of 20 identical pieces of paper. The pieces of paper are then put into a hat and mixed together. If a piece of paper is selected at random from the hat, what is the probability that the number on it is a multiple of 3 ?
Each of the whole numbers from 1 to 20 is written on one of 20 identical pieces of paper. The pieces of paper are then put into a hat and mixed together. If a piece of paper is selected at random from the hat, what is the probability that the number on it is a multiple of 3 ?
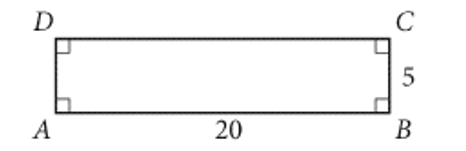
What is the ratio of AB to BC ?

What is the ratio of AB to BC ?
The curve models the number of fish in a certain population.
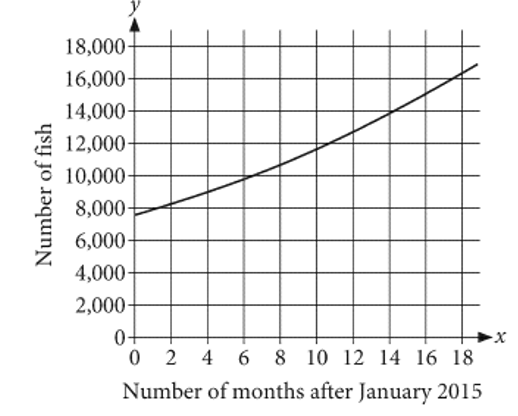
Which of the following is closest to the number of fish in the population 16 months after January 2015?
The curve models the number of fish in a certain population.

Which of the following is closest to the number of fish in the population 16 months after January 2015?
A length of 8 furlongs is equivalent to how many meters? (Use 1 furlong = 201 meters.)
A length of 8 furlongs is equivalent to how many meters? (Use 1 furlong = 201 meters.)
If 2x + 4 = 100, what is the value of 6x + 12 ?
If 2x + 4 = 100, what is the value of 6x + 12 ?
It takes 6 hours to travel m miles. At this rate, how much time, in hours, will it take to travel 5 m miles?
It takes 6 hours to travel m miles. At this rate, how much time, in hours, will it take to travel 5 m miles?
 The given function
The given function  models the number of flour beetles in a certain area, where
models the number of flour beetles in a certain area, where  represents the number of days after June 1. Which of the following is the best interpretation of the number 1.11 in this context?
represents the number of days after June 1. Which of the following is the best interpretation of the number 1.11 in this context?
 The given function
The given function  models the number of flour beetles in a certain area, where
models the number of flour beetles in a certain area, where  represents the number of days after June 1. Which of the following is the best interpretation of the number 1.11 in this context?
represents the number of days after June 1. Which of the following is the best interpretation of the number 1.11 in this context?
8x - 4y = 7
3x + 6y = 12
If (x, y) is the solution to the given system of equations, what is the value of x ?
8x - 4y = 7
3x + 6y = 12
If (x, y) is the solution to the given system of equations, what is the value of x ?
Albedos of Various Earth Surfaces

An albedo is the amount of light reflected from a surface divided by the amount of light falling on the surface. The amount is typically measured in watts per square meter. The table shows the minimum and maximum albedos for different types of surfaces on Earth.
In a desert region, the amount of sunlight falling on a surface with an area of one square meter is 1,000 watts. Which of the following could be the amount of sunlight, in watts, reflected from this one-square-meter surface?
Albedos of Various Earth Surfaces

An albedo is the amount of light reflected from a surface divided by the amount of light falling on the surface. The amount is typically measured in watts per square meter. The table shows the minimum and maximum albedos for different types of surfaces on Earth.
In a desert region, the amount of sunlight falling on a surface with an area of one square meter is 1,000 watts. Which of the following could be the amount of sunlight, in watts, reflected from this one-square-meter surface?
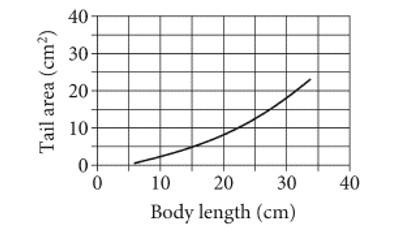
For a certain group of fish, the graph models the relationship between body length L, in centimeters (cm), and tail area A, in square centimeters  , where
, where  . Which equation represents the relationship between body length and tail area?
. Which equation represents the relationship between body length and tail area?
Solving quadratic equations using a graph is an effective method for locating estimated solutions or roots for quadratic equations or functions.
The real roots of a quadratic function might be zero, one (repeated), or two. Finding the origins involves solving a quadratic equation with the right-hand side equal to zero, such as ax² + bx + c = 0.
Points to consider to solve a quadratic equation using a graph :
• Rearrange the equation so that one side corresponds to the graphed function.
• Plot the function y= the other side of the equation.
• To find the solutions, draw vertical lines down to the x-axis from the intersection points.

For a certain group of fish, the graph models the relationship between body length L, in centimeters (cm), and tail area A, in square centimeters  , where
, where  . Which equation represents the relationship between body length and tail area?
. Which equation represents the relationship between body length and tail area?
Solving quadratic equations using a graph is an effective method for locating estimated solutions or roots for quadratic equations or functions.
The real roots of a quadratic function might be zero, one (repeated), or two. Finding the origins involves solving a quadratic equation with the right-hand side equal to zero, such as ax² + bx + c = 0.
Points to consider to solve a quadratic equation using a graph :
• Rearrange the equation so that one side corresponds to the graphed function.
• Plot the function y= the other side of the equation.
• To find the solutions, draw vertical lines down to the x-axis from the intersection points.




