Question
Three cyclic structures of monosaccharides are given below which of these are anomers
I) 
II) 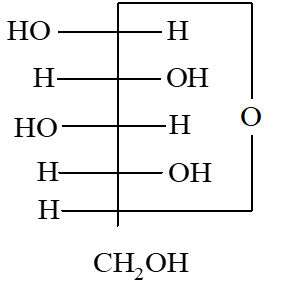
III) 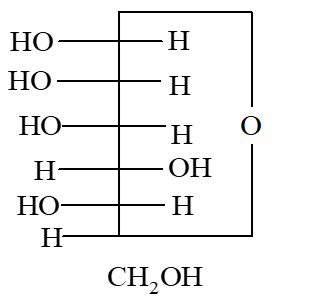
- I and II
- II and III
- I and III
- III is anomer of I and II
The correct answer is: I and II
Conceptual
Related Questions to study
In the following structure, anomeric carbon is

In the following structure, anomeric carbon is

Family  of curve represented by the differential equation of degree
of curve represented by the differential equation of degree
In this question, we have to find the degree of the differential equation. The degree is highest power or order of the derivative in the differential equation.
Family  of curve represented by the differential equation of degree
of curve represented by the differential equation of degree
In this question, we have to find the degree of the differential equation. The degree is highest power or order of the derivative in the differential equation.
The differential equation  (a is any constant) represents
(a is any constant) represents
The differential equation  (a is any constant) represents
(a is any constant) represents
Two parallel forces not having the same line of action form a couple if they are
In this question, we have to find the are two parallel forces not having the same line of action form a couple. A couple is a pair of forces, equal in magnitude, oppositely directed, and displaced by perpendicular distance or moment.
Two parallel forces not having the same line of action form a couple if they are
In this question, we have to find the are two parallel forces not having the same line of action form a couple. A couple is a pair of forces, equal in magnitude, oppositely directed, and displaced by perpendicular distance or moment.
Any two coplanar couples of equal moments
In this question, two coplanar couples of equal moments. Moments of couple is called torque. When two couples are present whose moments are equal and opposite then the forces are already balanced.
Any two coplanar couples of equal moments
In this question, two coplanar couples of equal moments. Moments of couple is called torque. When two couples are present whose moments are equal and opposite then the forces are already balanced.
Forces of magnitudes  and
and  act at a point parallel to the sides of an equilateral triangle taken in order. The resultant of these forces, is
act at a point parallel to the sides of an equilateral triangle taken in order. The resultant of these forces, is
Here we have to find the resultant force of the given magnitude. Firstly, find the component of the force which is Fsin θ and Fcos θ.
Forces of magnitudes  and
and  act at a point parallel to the sides of an equilateral triangle taken in order. The resultant of these forces, is
act at a point parallel to the sides of an equilateral triangle taken in order. The resultant of these forces, is
Here we have to find the resultant force of the given magnitude. Firstly, find the component of the force which is Fsin θ and Fcos θ.
If two forces  and
and  make an angle
make an angle 
 with each other and their resultant makes an angle
with each other and their resultant makes an angle  with the bisector of the angle between the two forces, then is equal to
with the bisector of the angle between the two forces, then is equal to
If two forces  and
and  make an angle
make an angle 
 with each other and their resultant makes an angle
with each other and their resultant makes an angle  with the bisector of the angle between the two forces, then is equal to
with the bisector of the angle between the two forces, then is equal to
If the square of the resultant of two equal forces is equal to  times their product, then the angle between the forces is
times their product, then the angle between the forces is
If the square of the resultant of two equal forces is equal to  times their product, then the angle between the forces is
times their product, then the angle between the forces is
The maximum resultant of two forces is P and the minimum resultant is  , the two forces are at right angles, the resultant is
, the two forces are at right angles, the resultant is
The maximum resultant of two forces is P and the minimum resultant is  , the two forces are at right angles, the resultant is
, the two forces are at right angles, the resultant is
The resultant of two forces  and
and  is of magnitude P. If the force
is of magnitude P. If the force  is doubled,
is doubled,  remaining unaltered, the new resultant will be
remaining unaltered, the new resultant will be
The resultant of two forces  and
and  is of magnitude P. If the force
is of magnitude P. If the force  is doubled,
is doubled,  remaining unaltered, the new resultant will be
remaining unaltered, the new resultant will be
The resultant of two forces 3P and 2P is R, if the first force is doubled, the resultant is also doubled. The angle between the forces is
In this question, we have to make two equations of Resultant as per given instruction and solve the equations and find the angle. Remember the formula of Resultant is R =
The resultant of two forces 3P and 2P is R, if the first force is doubled, the resultant is also doubled. The angle between the forces is
In this question, we have to make two equations of Resultant as per given instruction and solve the equations and find the angle. Remember the formula of Resultant is R =
If the gradient of the tangent at any point (x, y) of a curve which passes through the point  is
is  then equation of the curve is
then equation of the curve is
If the gradient of the tangent at any point (x, y) of a curve which passes through the point  is
is  then equation of the curve is
then equation of the curve is
If  , then
, then  is
is
In this question, we have given ycosx + xcosy =π . Differentiate this equation with request to x and do separate second derivate and find the value of it.
If  , then
, then  is
is
In this question, we have given ycosx + xcosy =π . Differentiate this equation with request to x and do separate second derivate and find the value of it.
The solution of the differential equation  is equal to
is equal to
In this question, you have to find the solution of differential equation x4 + x3 y + cosec (xy) = 0. Use variable separable method to find the solution.
The solution of the differential equation  is equal to
is equal to
In this question, you have to find the solution of differential equation x4 + x3 y + cosec (xy) = 0. Use variable separable method to find the solution.



